Advertisements
Advertisements
प्रश्न
A small bulb is placed at the bottom of a tank containing water to a depth of 80 cm. What is the area of the surface of water through which light from the bulb can emerge out? Refractive index of water is 1.33. (Consider the bulb to be a point source.)
उत्तर
Actual depth of the bulb in water, d1 = 80 cm = 0.8 m
Refractive index of water, μ = 1.33
The given situation is shown in the following figure:
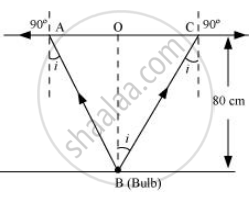
Where,
i = Angle of incidence
r = Angle of refraction = 90°
Since the bulb is a point source, the emergent light can be considered as a circle of radius, R = `"AC"/2` = AO = OB
Using Snell’ law, we can write the relation for the refractive index of water as:
μ = `(sin "r")/(sin "i")`
1.33 = `(sin 90°)/(sin "i")`
∴ i = `sin^-1 (1/1.33)` = 48.75°
Using the given figure, we have the relation:
tan i = `"OC"/"OB" = "R"/"d"_1`
∴ R = tan 48.75° × 0.8 = 0.91 m
∴ Area of the surface of water = πR2 = π (0.91)2 = 2.61 m2
Hence, the area of the surface of water through which the light from the bulb can emerge is approximately 2.61 m2.
APPEARS IN
संबंधित प्रश्न
Calculate the speed of light in a medium whose critical angle is 45°. Does critical angle for a given pair of media depend on the wavelength of incident light ? Give reason.
Use Huygens’ geometrical construction to show the propagation of plane wavefront a rarer medium (1) to a denser medium (2) undergoing refraction.
Hence derive Snell’s law of refraction.
Find a critical angle for glass and water pair, given the refractive index of glass, is 1 ·62 and that of water is 1 ·33.
Derive Snell’s law of refraction using Huygens’s wave theory.
According to Snell’s law, ______.
For small angles Snell’s law becomes ______.
Using Huygen’s wave theory of light, prove Snell’s law of refraction of light.
The mixture a pure liquid and a solution in a long vertical column (i.e, horizontal dimensions << vertical dimensions) produces diffusion of solute particles and hence a refractive index gradient along the vertical dimension. A ray of light entering the column at right angles to the vertical is deviated from its original path. Find the deviation in travelling a horizontal distance d << h, the height of the column.
If light passes near a massive object, the gravitational interaction causes a bending of the ray. This can be thought of as happening due to a change in the effective refractive index of the medium given by n(r) = 1 + 2 GM/rc2 where r is the distance of the point of consideration from the centre of the mass of the massive body, G is the universal gravitational constant, M the mass of the body and c the speed of light in vacuum. Considering a spherical object find the deviation of the ray from the original path as it grazes the object.
A ray of light is incident on a glass prism of refractive index µ and refracting angle A. If it just suffers total internal reflection at the other face, obtain a relation between the angle of incidence, angle of prism and critical angle.
