Advertisements
Advertisements
प्रश्न
Using opposite angles test for parallelogram, prove that every rectangle is a parallelogram.
उत्तर
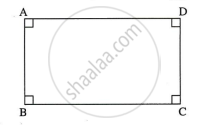
Given: `square`ABCD is a rectangle.
To prove: `square`ABCD is a parallelogram.
Proof:
`square`ABCD is a rectangle.
∠A = ∠B = ∠C = ∠D = 90° ...(angles of rectangle)
∠A = ∠B = ∠C = ∠D ...(each angle 90°)
A quadrilateral is a parallelogram if its pairs of opposite angles are congruent.
∴ `square`ABCD is a parallelogram.
APPEARS IN
संबंधित प्रश्न
In Fig. 17.29, suppose it is known that DE = DF. Then, is ΔABC isosceles? Why or why not?
Which of the following statement is true for a rectangle?
Its diagonals are equal and perpendicular, and bisect each other.
Fill in the blank of the following, so as to make the statement true:
A square is a rectangle in which .....
State with Reason Whether the Following Statement is ‘True’ Or ‘False’.
Every rectangle is a parallelogram.
State with reason whether the following statement is ‘true’ or ‘false’.
Every parallelogram is a rectangle.
Show that the bisectors of angles of a parallelogram form a rectangle
If the diagonals of a quadrilateral are equal and bisect each other, then the quadrilateral is a ______.
All squares are rectangles.
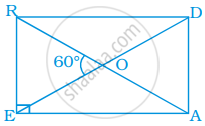
In rectangle READ, find ∠EAR, ∠RAD and ∠ROD
A rectangular MORE is shown below:
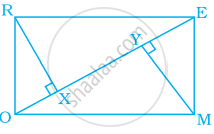
Answer the following questions by giving appropriate reason.
- Is RE = OM?
- Is ∠MYO = ∠RXE?
- Is ∠MOY = ∠REX?
- Is ΔMYO ≅ ΔRXE?
- Is MY = RX?
