Advertisements
Advertisements
प्रश्न
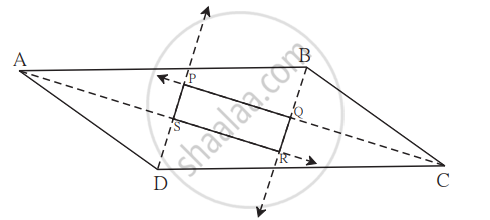
Show that the bisectors of angles of a parallelogram form a rectangle
उत्तर
Given: A parallelogram in which bisector of angle A, B, C, D intersect at P, Q, R, S to form a quadrilateral PQRS.
To prove: Quadrilateral PQRS is a rectangle.
Proof: Since ABCD is a parallelogram.
Therefore, AB || DC.
Now, AB || DC, and transversal AD cuts them, so we have
∠A + ∠D = 180°
`1/2 ∠"A" + 1/2 ∠ "D" = (180^circ)/2`
∠DAS + ∠ADS = 90°
But in ΔASD, we have
∠ADS + ∠DAS + ∠ASD = 180°
90° + ∠ASD = 180°
∠ASD = 90°
∠RSP = ∠ASD ...(vertically opposite angle)
∠RSP = 90°
Similarly, we can prove that
∠SRQ = 90°, ∠RQP = 90° and ∠QPS = 90°
Thus, PQRS is a quadrilateral each of whose angle is 90°.
Hence, PQRS is a rectangle.
APPEARS IN
संबंधित प्रश्न
Which of the following statement is true for a rectangle?
It has all its sides of equal length.
Which of the following statement true for a square?
Its diagonals bisect each other at right angle.
In a rectangle ABCD, prove that ∆ACB ≅ ∆CAD.
Find the length of the diagonal of a rectangle whose sides are 12 cm and 5 cm.
A mason has made a concrete slab. He needs it to be rectangular. In what different ways can he make sure that it is rectangular?
Prove that quadrilateral formed by the intersection of angle bisectors of all angles of a parallelogram is a rectangle.
Draw a rectangle ABCD such that l(AB) = 6.0 cm and l (BC) = 4.5 cm.
If diagonal of a rectangle is 26 cm and one side is 24 cm, find the other side.
A parallelogram PQRS is constructed with sides QR = 6 cm, PQ = 4 cm and ∠PQR = 90°. Then PQRS is a ______.
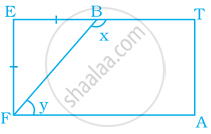
A playground is in the form of a rectangle ATEF. Two players are standing at the points F and B where EF = EB. Find the values of x and y.