Advertisements
Advertisements
प्रश्न
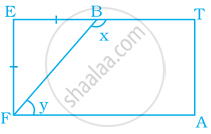
A playground is in the form of a rectangle ATEF. Two players are standing at the points F and B where EF = EB. Find the values of x and y.
उत्तर
Given, A rectangle ATEF in which EF = EB.
Then, ΔFEB is an isosceles triangle.
Therefore, by the angle sum property of a triangle, we have
∠EFB + ∠EBF + ∠FEB = 180° ...[Angle sum property of triangle]
⇒ ∠EFB + ∠EBF + 90° = 180° ...[∵ In a rectangle, each angle is of 90°]
⇒ 2∠EFB = 90° ...[∵ ∠EFB = ∠EBF]
∠EFB = 45° and ∠EBF = 45°
Now, ∠x = 180° – 45° = 135° ...[Linear pair]
And ∠EFB + ∠y = 90° ...[∵ In a rectangle, each angle is of 90°]
⇒ ∠y = 90° – 45° = 45°
APPEARS IN
संबंधित प्रश्न
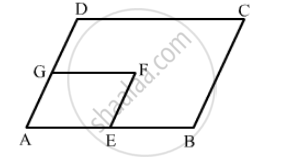
In the following figure, ABCD and AEFG are parallelograms. If ∠C = 55°, what is the measure of ∠F?
Which of the following statement is true for a rectangle?
It has two pairs of equal sides.
Fill in the blank in the following, so as to make the statement true:
A rectangle is a parallelogram in which .....
Fill in the blank of the following, so as to make the statement true:
A square is a rectangle in which .....
A window frame has one diagonal longer than the other. Is the window frame a rectangle? Why or why not?
If diagonal of a rectangle is 26 cm and one side is 24 cm, find the other side.
Show that the bisectors of angles of a parallelogram form a rectangle
Rectangle is a regular quadrilateral.
Every trapezium is a rectangle.
A rectangular MORE is shown below:
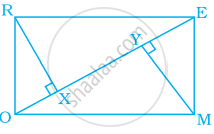
Answer the following questions by giving appropriate reason.
- Is RE = OM?
- Is ∠MYO = ∠RXE?
- Is ∠MOY = ∠REX?
- Is ΔMYO ≅ ΔRXE?
- Is MY = RX?
