Question

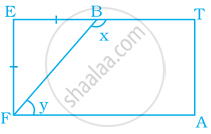
A playground is in the form of a rectangle ATEF. Two players are standing at the points F and B where EF = EB. Find the values of x and y.

Answer 1

Given, A rectangle ATEF in which EF = EB.

Then, ΔFEB is an isosceles triangle.

Therefore, by the angle sum property of a triangle, we have

∠EFB + ∠EBF + ∠FEB = 180°   ...[Angle sum property of triangle]

⇒ ∠EFB + ∠EBF + 90° = 180°   ...[∵ In a rectangle, each angle is of 90°]

⇒ 2∠EFB = 90°   ...[∵ ∠EFB = ∠EBF]

∠EFB = 45° and ∠EBF = 45°

Now, ∠x = 180° – 45° = 135°   ...[Linear pair] 

And ∠EFB + ∠y = 90°   ...[∵ In a rectangle, each angle is of 90°]

⇒ ∠y = 90° – 45° = 45°