Question

In a rectangle ABCD, AB = 25 cm and BC = 15. In what ratio does the bisector of ∠C divide AB?

Answer 1

Given, AB = 25 cm and BC = 15 cm

Now, In rectangle ABCD,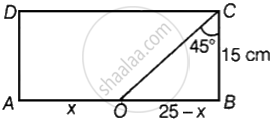

CO is the bisector of ∠C and it divides AB.

∴ ∠OCB = ∠OCD = 45°

∴ ∠OCB = ∠OCD = 45°

In ΔOCB, we have

∠CBO + ∠OCB + ∠COB = 180°   ...[Angle sum property of triangle]

90° + 45° + ∠COB = 180°

∠COB = 180° – 90° + 45°

∠COB = 180° – 135° = 45°

Now, In ΔOCB,

∠OCB = ∠COB

Then, OB = OC

⇒ OB = 15 cm

CO divides AB in the ratio AO : OB

Let AO be x, then OB = AB – x = 25 – x

Hence, AO : OB = x : 25 – x

⇒ 10 : 15

⇒ 2 : 3