Question

Prove that quadrilateral formed by the intersection of angle bisectors of all angles of a parallelogram is a rectangle.

Answer 1

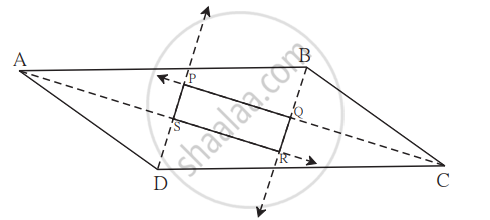
Given: `square`ABCD is a parallelogram.

To prove: `square`PQRS is a rectangle.

Proof:

`square`ABCD is a parallelogram.      ...(Given)

∠ADC + ∠BCD = 180°      ...(Adjacent angles of a parallelogram are supplementary.)

Multiplying each side by `1/2`,

`1/2` ∠ADC + `1/2` ∠BCD = `1/2xx180°`      ...(i)

But,

`1/2` ∠ADC = ∠PDC      ...(Ray DP bisects ∠ADC)    ...(ii)

And `1/2` ∠BCD = ∠PCD     ...(Ray CP bisects ∠BCD)    ...(iii)

∴ ∠PDC + ∠PCD = 90°      ...[From (i), (ii) and (iii)]     ...(iv)

In ΔPDC,

∠PDC + ∠PCD + ∠DPC = 180°      ...(The sum of the measures of the three angles of a triangle is 180°.)

∴ 90° + ∠DPC = 180°       ...[from (iv)]

∴ ∠DPC = 180° – 90°

∴ ∠DPC = 90°

That means ∠SPQ = 90°     ...(D-S-P, P-Q-C)    ...(v)

Similarly, we can prove that, ∠SRQ = 90°     ...(vi)

Similarly, ∠ASD = 90° and ∠BQC = 90°     ...(vii)

∠PSR = ∠ASD     ...(vertex angle)

∴ ∠PSR = 90°     ...[From (vii)]    ...(viii)

Similarly, ∠PQR = 90°     ...(ix)

In `square`PQRS,

∠SPQ = ∠SRQ = ∠PSR = ∠PQR = 90°     ...[From (v), (vi), (viii) and (ix)]

∴ `square`PQRS is a rectangle.