Advertisements
Advertisements
प्रश्न
In the given figure, if points P, Q, R, S are on the sides of parallelogram such that AP = BQ = CR = DS then prove that `square`PQRS is a parallelogram.
Advertisements
उत्तर
Given: `square` ABCD is a parallelogram.
AP = BQ = CR = DS
To prove: `square`PQRS is a parallelogram.
Proof:
AP = CR ...(Given) ...(i)
`square`ABCD is a parallelogram.
AB = CD ...(opposite sides of parallelogram)
∴ AP + PB = CR + RD ...[A-P-B, D-R-C] ...(ii)
∴ PB = RD ...[From (i) and (ii)] ...(iii)
∠ABC ≅ ∠ADC ...(Opposite angles of parallelogram)
That is, ∠PBQ ≅ ∠RDS ...(A-P-B, B-Q-C, C-R-D and A-S-D) ...(iv)
In ΔPBQ and ΔRDS,
Seg PB ≅ Seg RD ...[From (iii)]
∠PBQ ≅ ∠RDS ...[From (iv)]
Seg BQ ≅ Seg SD ...(Given)
∴ ΔPBQ ≅ ΔRDS ...[SAS test]
Seg PQ ≅ Seg RS ...(c.s.c.t) ...(v)
Thus, we can prove that, ΔPAS ≅ ΔRCQ,
∴ Seg PS ≅ Seg RQ ...(vi)
In `square`PQRS,
Seg PQ ≅ Seg RS ...[From (v)]
Seg PS ≅ Seg RQ ...[from (vi)]
If the opposite sides of a quadrilateral are congruent, then it is a parallelogram.
∴ `square`PQRS is a parallelogram.
APPEARS IN
संबंधित प्रश्न
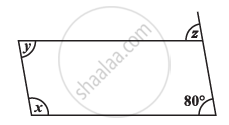
Consider the given parallelograms. Find the values of the unknowns x, y, z.
Can a quadrilateral ABCD be a parallelogram if ∠D + ∠B = 180°?
The adjacent figure HOPE is a parallelogram. Find the angle measures x, y and z. State the properties you use to find them.
In the given figure, `square`PQRS and `square`ABCR are two parallelograms. If ∠P = 110° then find the measures of all angles of `square`ABCR.
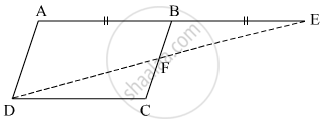
In the given figure, `square`ABCD is a parallelogram. Point E is on the ray AB such that BE = AB then prove that line ED bisects seg BC at point F.
Construct ☐ PQRS, such that l(PQ) = 3.5 cm, l(QR) = 5.6 cm, l(RS) = 3.5 cm, m∠Q = 110°, m∠R = 70°. If it is given that ☐ PQRS is a parallelogram, which of the given information is unnecessary?
Construct a parallelogram ABCD such that l(BC) = 7 cm, m∠ABC = 40° , l(AB) = 3 cm.
In parallelogram PQRS, ∠Q = (4x – 5)° and ∠S = (3x + 10)°. Calculate: ∠Q and ∠R.
In parallelogram ABCD, X and Y are midpoints of opposite sides AB and DC respectively. Prove that:
(i) AX = YC
(ii) AX is parallel to YC
(iii) AXCY is a parallelogram.
The given figure shows parallelogram ABCD. Points M and N lie in diagonal BD such that DM = BN.
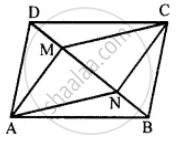
Prove that:
(i) ∆DMC = ∆BNA and so CM = AN
(ii) ∆AMD = ∆CNB and so AM CN
(iii) ANCM is a parallelogram.
Iron rods a, b, c, d, e, and f are making a design in a bridge as shown in the figure. If a || b, c || d, e || f, find the marked angles between b and c
Iron rods a, b, c, d, e, and f are making a design in a bridge as shown in the figure. If a || b, c || d, e || f, find the marked angles between d and e
Which of the following is a property of a parallelogram?
The angle between the two altitudes of a parallelogram through the same vertex of an obtuse angle of the parallelogram is 30°. The measure of the obtuse angle is ______.
ABCD is a parallelogram. Points P and Q are taken on the sides AB and AD respectively and the parallelogram PRQA is formed. If ∠C = 45°, find ∠R.
In parallelogram ABCD, the angle bisector of ∠A bisects BC. Will angle bisector of B also bisect AD? Give reason.
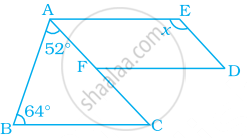
In the following figure, FD || BC || AE and AC || ED. Find the value of x.
Construct a parallelogram HOME with HO = 6 cm, HE = 4 cm and OE = 3 cm.
The following figure RUNS is parallelogram. Find x and y. (Lengths are in cm)
