Advertisements
Advertisements
प्रश्न
Which of the following statements are true (T) and which are false (F):
The bisectors of two equal angles of a triangle are equal
उत्तर
True (T)
Reason: Since it an isosceles triangle, the lengths of bisectors of the two equal angles are equal
APPEARS IN
संबंधित प्रश्न
Prove that each angle of an equilateral triangle is 60°.
Angles A, B, C of a triangle ABC are equal to each other. Prove that ΔABC is equilateral.
In a ΔPQR, if PQ = QR and L, M and N are the mid-points of the sides PQ, QR and RP
respectively. Prove that LN = MN.
Which of the following statements are true (T) and which are false (F):
The measure of each angle of an equilateral triangle is 60°
Fill the blank in the following so that the following statement is true.
In right triangles ABC and DEF, if hypotenuse AB = EF and side AC = DE, then ΔABC ≅ Δ ……
In ΔABC, if ∠A = 40° and ∠B = 60°. Determine the longest and shortest sides of the triangle.
Which of the following statements are true (T) and which are false (F)?
Sum of any two sides of a triangle is greater than twice the median drawn to the third side.
In ∆PQR, if ∠R > ∠Q, then ______.
In the following figure, D and E are points on side BC of a ∆ABC such that BD = CE and AD = AE. Show that ∆ABD ≅ ∆ACE.
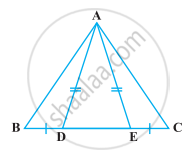
ABC is an isosceles triangle with AB = AC and D is a point on BC such that AD ⊥ BC (Figure). To prove that ∠BAD = ∠CAD, a student proceeded as follows:

In ∆ABD and ∆ACD,
AB = AC (Given)
∠B = ∠C (Because AB = AC)
and ∠ADB = ∠ADC
Therefore, ∆ABD ≅ ∆ACD (AAS)
So, ∠BAD = ∠CAD (CPCT)
What is the defect in the above arguments?
[Hint: Recall how ∠B = ∠C is proved when AB = AC].
