Advertisements
Advertisements
प्रश्न
Which of the following statements are true (T) and which are false (F):
The bisectors of two equal angles of a triangle are equal
उत्तर
True (T)
Reason: Since it an isosceles triangle, the lengths of bisectors of the two equal angles are equal
APPEARS IN
संबंधित प्रश्न
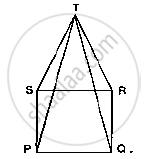
In Fig. 10.23, PQRS is a square and SRT is an equilateral triangle. Prove that
(i) PT = QT (ii) ∠TQR = 15°
The vertical angle of an isosceles triangle is 100°. Find its base angles.
In a ΔABC, it is given that AB = AC and the bisectors of ∠B and ∠C intersect at O. If M is a point on BO produced, prove that ∠MOC = ∠ABC.
Prove that each angle of an equilateral triangle is 60°.
ABC is a right angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
ABC is a triangle. The bisector of the exterior angle at B and the bisector of ∠C intersect each other at D. Prove that ∠D = \[\frac{1}{2}\] ∠A.
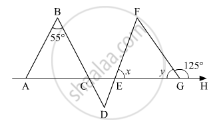
In the given figure, if AB || DE and BD || FG such that ∠FGH = 125° and ∠B = 55°, find x and y.
In a ΔABC, if ∠A = 60°, ∠B = 80° and the bisectors of ∠B and ∠C meet at O, then ∠BOC =
In the given figure, what is y in terms of x?

In ∆PQR, ∠R = ∠P and QR = 4 cm and PR = 5 cm. Then the length of PQ is ______.
