Advertisements
Advertisements
प्रश्न
ABC is a triangle. The bisector of the exterior angle at B and the bisector of ∠C intersect each other at D. Prove that ∠D = \[\frac{1}{2}\] ∠A.
उत्तर
In the given ΔABC, the bisectors of ext,∠B and ∠Cntersect at D
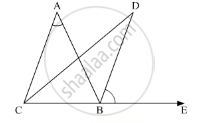
We need to prove: `∠D = 1/2 ∠A`
Now, using the exterior angle theorem,
\[\angle ABE = \angle BAC + \angle ACB\] .….(1)
\[As \angle \text {ABE and } \angle \text { ACB are bisected }\]
\[\angle DCB = \frac{1}{2}\angle ACB\]
Also,
\[\angle DBA = \frac{1}{2}\angle ABE\]
Further, applying angle sum property of the triangle
In ΔDCB
\[\angle CDB + \angle DCB + \angle CBD = 180^\circ\]
\[ \Rightarrow \angle CDB + \frac{1}{2}\angle ACB + \left( \angle DBA + \angle ABC \right) = 180^\circ\]
\[\angle CDB + \frac{1}{2}\angle ACB + \left( \frac{1}{2}\angle ABE + \angle ABC \right) = 180^\circ . . . . . \left( 2 \right)\]
Also, CBE is a straight line, So, using linear pair property
\[\Rightarrow \angle ABC + \angle ABE = 180^\circ\]
\[ \Rightarrow \angle ABC + \frac{1}{2}\angle ABE + \frac{1}{2}\angle ABE = 180^\circ \]
\[ \Rightarrow \angle ABC + \frac{1}{2}\angle ABE = 180^\circ - \frac{1}{2}\angle ABE . . . . . \left( 3 \right)\]
So, using (3) in (2)
\[\angle CDB + \frac{1}{2}\angle ACB + \left( 180^\circ - \frac{1}{2}\angle ABE \right) = 180^\circ \]
\[ \Rightarrow \angle CDB + \frac{1}{2}\angle ACB - \frac{1}{2}\angle ABE = 0\]
\[ \Rightarrow \angle CDB = \frac{1}{2}\left( \angle ABE - \angle ACB \right)\]
\[ \Rightarrow \angle CDB = \frac{1}{2}\angle CAB\]
\[ \Rightarrow \angle D = \frac{1}{2}\angle A\]
Hence proved.
APPEARS IN
संबंधित प्रश्न
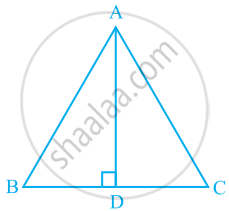
In ΔABC, AD is the perpendicular bisector of BC (see the given figure). Show that ΔABC is an isosceles triangle in which AB = AC.
In a ΔABC, if ∠A=l20° and AB = AC. Find ∠B and ∠C.
If the base of an isosceles triangle is produced on both sides, prove that the exterior angles so formed are equal to each other.
Determine the measure of each of the equal angles of a right-angled isosceles triangle.
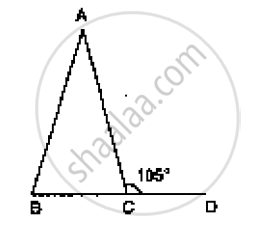
In Figure 10.24, AB = AC and ∠ACD =105°, find ∠BAC.
Which of the following statements are true (T) and which are false (F):
The measure of each angle of an equilateral triangle is 60°
In a ΔABC, if ∠B = ∠C = 45°, which is the longest side?
Fill in the blank to make the following statement true.
The sum of three altitudes of a triangle is ..... than its perimeter.
In the given figure, what is the value of x?
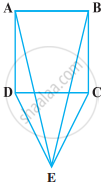
CDE is an equilateral triangle formed on a side CD of a square ABCD (Figure). Show that ∆ADE ≅ ∆BCE.