Advertisements
Advertisements
प्रश्न
Why is the diffraction of sound waves more evident in daily experience than that of light wave?
उत्तर
The frequencies of sound waves lie between 20 Hz to 20 kHz, their wavelength ranges between 15 m to 15 mm. The diffraction occurs if the wavelength of waves is nearly equal to slit width.
The wavelength of light waves is 7000 × 10–10 m to 4000 × 10–10 m. For observing diffraction of light we need a very narrow slit width. In daily life experience, we observe the slit width very near to the wavelength of sound waves as compared to light waves. Thus, the diffraction of sound waves is more evident in daily life than that of light waves.
APPEARS IN
संबंधित प्रश्न
Show that the fringe pattern on the screen is actually a superposition of slit diffraction from each slit.
In Young’s double slit experiment using monochromatic light of wavelength λ, the intensity of light at a point on the screen where path difference is λ, is K units. Find out the intensity of light at a point where path difference is λ/3.
Suppose white light falls on a double slit but one slit is covered by a violet filter (allowing λ = 400 nm). Describe the nature of the fringe pattern observed.
A plate of thickness t made of a material of refractive index µ is placed in front of one of the slits in a double slit experiment. (a) Find the change in the optical path due to introduction of the plate. (b) What should be the minimum thickness t which will make the intensity at the centre of the fringe pattern zero? Wavelength of the light used is \[\lambda.\] Neglect any absorption of light in the plate.
Wavefront is ______.
The force required to double the length of a steel wire of area 1 cm2, if its Young's modulus Y= 2 × 1011/m2 is:
Two balls are projected at an angle θ and (90° − θ) to the horizontal with the same speed. The ratio of their maximum vertical heights is:
Consider a two-slit interference arrangement (Figure) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of λ such that the first minima on the screen falls at a distance D from the centre O.
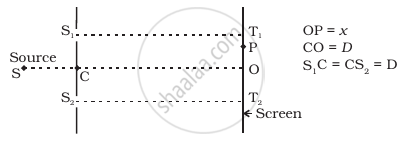
How will the interference pattern in Young's double-slit experiment be affected if the source slit is moved away from the plane of the slits?
How will the interference pattern in Young's double-slit experiment be affected if the phase difference between the light waves emanating from the two slits S1 and S2 changes from 0 to π and remains constant?
