Advertisements
Advertisements
प्रश्न
How will the interference pattern in Young's double-slit experiment be affected if the phase difference between the light waves emanating from the two slits S1 and S2 changes from 0 to π and remains constant?
उत्तर
Fringe width = β = `"Dλ"/"d"`
If the phase difference becomes π, then the central fringe will be a dark fringe, since
I = `4"I"_0 cos^2 θ/2`
= `4"I"_0 cos^2 π/2`
= 0
APPEARS IN
संबंधित प्रश्न
Show that the fringe pattern on the screen is actually a superposition of slit diffraction from each slit.
A parallel beam of light of wavelength 500 nm falls on a narrow slit and the resulting diffraction pattern is observed on a screen 1 m away. It is observed that the first minimum is a distance of 2.5 mm away from the centre. Find the width of the slit.
White light is used in a Young's double slit experiment. Find the minimum order of the violet fringe \[\left( \lambda = 400\text{ nm} \right)\] which overlaps with a red fringe \[\left( \lambda = 700\text{ nm} \right).\]
A double slit S1 − S2 is illuminated by a coherent light of wavelength \[\lambda.\] The slits are separated by a distance d. A plane mirror is placed in front of the double slit at a distance D1 from it and a screen ∑ is placed behind the double slit at a distance D2 from it (see the following figure). The screen ∑ receives only the light reflected by the mirror. Find the fringe-width of the interference pattern on the screen.
In a Young's double slit experiment, \[\lambda = 500\text{ nm, d = 1.0 mm and D = 1.0 m.}\] Find the minimum distance from the central maximum for which the intensity is half of the maximum intensity.
Draw the intensity distribution as function of phase angle when diffraction of light takes place through coherently illuminated single slit.
Young's double slit experiment is made in a liquid. The 10th bright fringe lies in liquid where 6th dark fringe lies in vacuum. The refractive index of the liquid is approximately
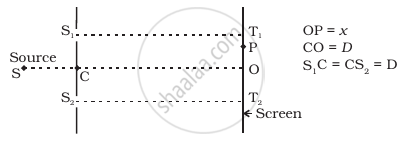
Consider a two-slit interference arrangement (Figure) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of λ such that the first minima on the screen falls at a distance D from the centre O.
In a Young's double slit experiment, the width of the one of the slit is three times the other slit. The amplitude of the light coming from a slit is proportional to the slit- width. Find the ratio of the maximum to the minimum intensity in the interference pattern.
In Young's double-slit experiment, the screen is moved away from the plane of the slits. What will be its effect on the following?
- The angular separation of the fringes.
- Fringe-width.
