Advertisements
Advertisements
Question
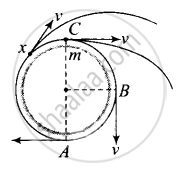
A bob of mass m suspended by a light string of length L is whirled into a vertical circle as shown in figure. What will be the trajectory of the particle if the string is cut at
- Point B?
- Point C?
- Point X?

Solution
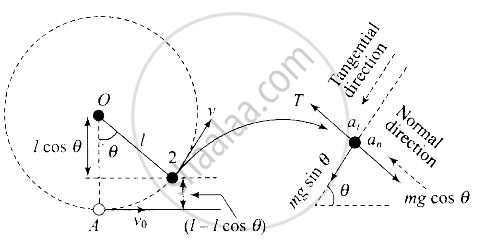
According to the situation shown above when a bob of mass m is whirled into a vertical circle, the required centripetal force is obtained from the net force towards the center at any point of time in the string. Tension in the string is variable and it is always towards center. But the gravitational force on the bob is always towards center. The speed of the body will be different at different points. So the equations of dynamical equilibrium (Fc = mac, Ft = mat) must be satisfied at all the points. Let when the string makes an angle of 9 with vertical, the speed of mass is v.
Apply Newton’s law perpendicular to the string:
Mg sin = ma, ⇒ a,= g sin
The above equation gives tangential acceleration as a function of the angle. At lowest point = 0° and at highest point = 180°. So at both points sin 9 = 0. Hence a, = 0 at both points L and H.
At point M, = 90°, then a1 = g. It is the maximum value of at
Apply Newton’s law along the string: T – mg cos = mac
or T = mgcos + mv2/r ......(i)
As the body goes up, its velocity will go on decreasing and angle θ will go on increasing. The maximum speed of the body will be at the lowest point L and minimum at the highest point H. Then from the above relation we can find that tension will be maximum at the lowest point and minimum at the highest point.
Tension at the lowest point (θ – 0°, v = vL): TL = `mg + m v_L^2/r` ......(ii)
Tension at the highest point (θ – 180°, v = vH): TH = `mg + m v_H^2/r` ......(iii)
When a string is cut, the tension in string becomes zero and centripetal force is not provided. Hence, the bob tends to move in along the direction of its velocity.
- If the string is cut at any point, then velocity of the body of mass m is along the tangent to the circle. A tangent at point B is vertically downward so the trajectory of the particle is a straight line.
- The tangent at point C is horizontally towards the right.
So the trajectory of the particle is the parabola. - The tangent at point X makes some angle with the horizontal. Again bob will follow a parabolic path with a vertex higher than C.
APPEARS IN
RELATED QUESTIONS
A body is initially at rest. It undergoes one-dimensional motion with constant acceleration. The power delivered to it at time t is proportional to ______.
A heavy stone is thrown from a cliff of height h with a speed v. The stoen will hit the ground with maximum speed if it is thrown
A heavy stone is thrown in from a cliff of height h in a given direction. The speed with which it hits the ground
(a) must depend on the speed of projection
(b) must be larger than the speed of projection
(c) must be independent of the speed of projection
(d) may be smaller than the speed of projection.
You lift a suitcase from the floor and keep it on a table. The work done by you on the suitcase does not depend on
(a) the path taken by the suitcase
(b) the time taken by you in doing so
(c) the weight of the suitcase
(d) your weight
Figure shows a particle sliding on a frictionless track which terminates in a straight horizontal section. If the particle starts slipping from point A, how far away from the track will the particle hit the ground?

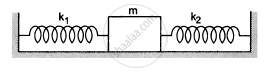
A block of mass m is attached to two unstretched springs of spring constants k1 and k2 as shown in the following figure. The block is displaced towards the right through a distance x and is released. Find the speed of the block as it passes through the mean position shown.
In the following figure shows two blocks A and B, each of mass of 320 g connected by a light string passing over a smooth light pulley. The horizontal surface on which the block Acan slide is smooth. Block A is attached to a spring of spring constant 40 N/m whose other end is fixed to a support 40 cm above the horizontal surface. Initially, the spring is vertical and unstretched when the system is released to move. Find the velocity of the block A at the instant it breaks off the surface below it. Take g = 10 m/s2.
Figure following shows a light rod of length l rigidly attached to a small heavy block at one end and a hook at the other end. The system is released from rest with the rod in a horizontal position. There is a fixed smooth ring at a depth h below the initial position of the hook and the hook gets into the ring as it reaches there. What should be the minimum value of h so that the block moves in a complete circle about the ring?

A body is falling freely under the action of gravity alone in vacuum. Which of the following quantities remain constant during the fall?
Why is electrical power required at all when the elevator is descending? Why should there be a limit on the number of passengers in this case?
