Advertisements
Advertisements
Question
A car is moving with a uniform velocity 30 ms-1. It is stopped in 2 s by applying a force of 1500 N through its brakes. Calculate the following values : The change in momentum of car.
Solution
Initial velocity, u = 30 m/s
Final velocity, v = 0
Time, t = 2s
Force, F = 1500 N
Here, a = (v - u)/t = (0 - 30)/ 2 = - 15 ms-2. Here, negative sign indicates retardation.
Now, F = ma.
Or, m = F/a = (1500/ 15) = 100 kg.
Change in momentum = Final momentum - Initial momentum
Or, Δp = m (v - u)
Or, Δp = 100 (0 - 30)
Or, Δp = 3000 kg m/s-1
APPEARS IN
RELATED QUESTIONS
Suppose the ceiling in the previous problem is that of an elevator which is going up with an acceleration of 2.0 m/s2. Find the elongation.
Consider the situation shown in the following figure All the surfaces are frictionless and the string and the pulley are light. Find the magnitude of acceleration of the two blocks.

In the previous problem, suppose m2 = 2.0 kg and m3 = 3.0 kg. What should be the mass m, so that it remains at rest?
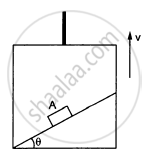
A block A can slide on a frictionless incline of angle θ and length l, kept inside an elevator going up with uniform velocity v in the following figure. Find the time taken by the block to slide down the length of the incline if it is released from the top of the incline.
Two bodies A and B of same mass are moving with velocities v and 2v, respectively. Compare their (i) inertia and (ii) momentum.
How can Newton's first law of motion be obtained from the second law of motion?
Use Newton's second law to explain the following:
We always prefer to land on sand instead of hard floor while taking a high jump.
A stone is thrown vertically upward with a velocity of 9.8 m/s. When will it reach the ground?
The motion of a particle of mass m is given by x = 0 for t < 0 s, x(t) = A sin 4 pt for 0 < t < (1/4) s (A > o), and x = 0 for t > (1/4) s. Which of the following statements is true?
- The force at t = (1/8) s on the particle is – 16π2 Am.
- The particle is acted upon by on impulse of magnitude 4π2 A m at t = 0 s and t = (1/4) s.
- The particle is not acted upon by any force.
- The particle is not acted upon by a constant force.
- There is no impulse acting on the particle.
Why does a child feel more pain when she falls down on a hard cement floor, than when she falls on the soft muddy ground in the garden?
