Advertisements
Advertisements
Question
A copper wire of cross-sectional area 0.01 cm2 is under a tension of 20N. Find the decrease in the cross-sectional area. Young modulus of copper = 1.1 × 1011 N m−2 and Poisson ratio = 0.32.
`["Hint" : (Delta"A")/"A"=2(Delta"r")/"r"]`
Solution
Given:
Cross-sectional area of copper wire A = 0.01 cm2 = 10−6 m2
Applied tension T = 20 N
Young modulus of copper Y = 1.1 × 1011 N/m2
Poisson ratio σ = 0.32
We know that: \[Y = \frac{FL}{A ∆ L}\]
\[\Rightarrow \frac{∆ L}{L} = \frac{F}{AY}\]
\[ = \frac{20}{{10}^{- 6} \times 1 . 1 \times {10}^{11}} = 18 . 18 \times {10}^{- 5} \]
\[\text{ Poisson's ratio }, \sigma = \frac{\frac{∆ d}{d}}{\frac{∆ L}{L}} = 0 . 32\]
\[\text{ Where d is the transverse length }\]
\[\text{ So }, \frac{∆ d}{d} = \left( 0 . 32 \right) \times \frac{∆ L}{L}\]
\[ = 0 . 32 \times \left( 18 . 18 \right) \times {10}^{- 5} = 5 . 81 \times {10}^{- 5} \]
\[\text{ Again }, \frac{∆ A}{A} = \frac{2 ∆ r}{r} = \frac{2 ∆ d}{d}\]
\[ \Rightarrow ∆ A = \frac{2 ∆ d}{d}A\]
\[ \Rightarrow ∆ A = 2 \times \left( 5 . 8 \times {10}^{- 5} \right) \times \left( 0 . 01 \right)\]
\[ = 1 . 164 \times {10}^{- 6} {\text{ cm }}^2\]
Hence, the required decrease in the cross -sectional area is \[1 . 164 \times {10}^{- 6} {\text{ cm } }^2\]
APPEARS IN
RELATED QUESTIONS
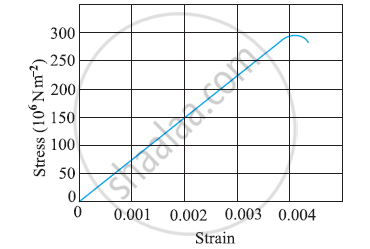
The figure shows the strain-stress curve for a given material. What are (a) Young’s modulus and (b) approximate yield strength for this material?
Two wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in Fig. 9.13. The unloaded length of steel wire is 1.5 m and that of brass wire is 1.0 m. Compute the elongations of the steel and the brass wires.

A 14.5 kg mass, fastened to the end of a steel wire of unstretched length 1.0 m, is whirled in a vertical circle with an angular velocity of 2 rev/s at the bottom of the circle. The cross-sectional area of the wire is 0.065 cm2. Calculate the elongation of the wire when the mass is at the lowest point of its path.
A steel rod of cross-sectional area 4 cm2 and 2 m shrinks by 0.1 cm as the temperature decreases in night. If the rod is clamped at both ends during the day hours, find the tension developed in it during night hours. Young modulus of steel = 1.9 × 1011 N m−2.
Young's modulus of a perfectly rigid body is ______.
The temperature of a wire is doubled. The Young’s modulus of elasticity ______.
The temperature of a wire is doubled. The Young’s modulus of elasticity ______.
A rigid bar of mass M is supported symmetrically by three wires each of length l. Those at each end are of copper and the middle one is of iron. The ratio of their diameters, if each is to have the same tension, is equal to ______.
What is the Young’s modulus for a perfect rigid body ?
A steel rod (Y = 2.0 × 1011 Nm–2; and α = 10–50 C–1) of length 1 m and area of cross-section 1 cm2 is heated from 0°C to 200°C, without being allowed to extend or bend. What is the tension produced in the rod?
A steel wire of mass µ per unit length with a circular cross section has a radius of 0.1 cm. The wire is of length 10 m when measured lying horizontal, and hangs from a hook on the wall. A mass of 25 kg is hung from the free end of the wire. Assuming the wire to be uniform and lateral strains << longitudinal strains, find the extension in the length of the wire. The density of steel is 7860 kg m–3 (Young’s modules Y = 2 × 1011 Nm–2).
If the yield strength of steel is 2.5 × 108 Nm–2, what is the maximum weight that can be hung at the lower end of the wire?
In nature, the failure of structural members usually result from large torque because of twisting or bending rather than due to tensile or compressive strains. This process of structural breakdown is called buckling and in cases of tall cylindrical structures like trees, the torque is caused by its own weight bending the structure. Thus the vertical through the centre of gravity does not fall within the base. The elastic torque caused because of this bending about the central axis of the tree is given by `(Ypir^4)/(4R) . Y` is the Young’s modulus, r is the radius of the trunk and R is the radius of curvature of the bent surface along the height of the tree containing the centre of gravity (the neutral surface). Estimate the critical height of a tree for a given radius of the trunk.
If Y, K and η are the values of Young's modulus, bulk modulus and modulus of rigidity of any material respectively. Choose the correct relation for these parameters.
A uniform metal rod of 2 mm2 cross section is heated from 0°C to 20°C. The coefficient of linear expansion of the rod is 12 × 10-6/°C, it's Young's modulus is 1011 N/m2. The energy stored per unit volume of the rod is ______.
The force required to stretch a wire of cross section 1 cm2 to double its length will be ______.
(Given Young's modulus of the wire = 2 × 1011 N/m2)
