Advertisements
Advertisements
Question
The temperature of a wire is doubled. The Young’s modulus of elasticity ______.
Options
will also double.
will become four times.
will remain same.
will decrease.
Solution
The temperature of a wire is doubled. The Young’s modulus of elasticity will decrease.
Explanation:
Young's modulus (Y): It is defined as the ratio of normal stress to longitudinal strain within the limit of proportionality.
`Y = "Normal stress"/"Longitudinal strain"`
= `(F/A)/((ΔL)/L)`
= `(FL)/(AΔL)`
The fractional change in length of any material is defined as `(ΔL)/L_0 = αΔT` where ΔT is the change in the temperature, L0 is the original length, α is the coefficient of linear expansion of the given material and L0 is the original length of material.
So, simply a change in length is due to change in temperature.
`ΔL = L_0αΔT`
And Young's modules
(Y) = `"Stress"/"Strain"`
= `(FL_0)/(A xx ΔL)`
= `(FL_0)/(AL_0 ΔT) ∝ 1/(ΔT)`
As Y ∝ 1/∆T
When temperature increases ∆T increases, hence Y decreases.
APPEARS IN
RELATED QUESTIONS
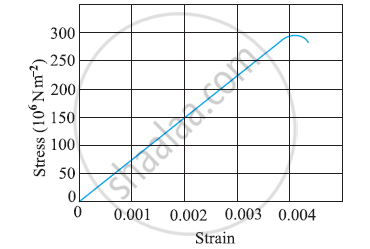
The figure shows the strain-stress curve for a given material. What are (a) Young’s modulus and (b) approximate yield strength for this material?
Read the following statements below carefully and state, with reasons, if it is true or false
The Young’s modulus of rubber is greater than that of steel;
The temperature of a wire is doubled. The Young’s modulus of elasticity ______.
Identical springs of steel and copper are equally stretched. On which, more work will have to be done?
A steel wire of mass µ per unit length with a circular cross section has a radius of 0.1 cm. The wire is of length 10 m when measured lying horizontal, and hangs from a hook on the wall. A mass of 25 kg is hung from the free end of the wire. Assuming the wire to be uniform and lateral strains << longitudinal strains, find the extension in the length of the wire. The density of steel is 7860 kg m–3 (Young’s modules Y = 2 × 1011 Nm–2).
If the yield strength of steel is 2.5 × 108 Nm–2, what is the maximum weight that can be hung at the lower end of the wire?
A steel rod of length 2l, cross sectional area A and mass M is set rotating in a horizontal plane about an axis passing through the centre. If Y is the Young’s modulus for steel, find the extension in the length of the rod. (Assume the rod is uniform.)
A metal wire of length L, area of cross section A and Young's modulus Y behaves as a spring of spring constant k given by:
A boy's catapult is made of rubber cord which is 42 cm long, with a 6 mm diameter of cross-section and negligible mass. The boy keeps a stone weighing 0.02 kg on it and stretches the cord by 20 cm by applying a constant force. When released, the stone flies off with a velocity of 20 ms-1. Neglect the change in the area of the cross-section of the cord while stretched. Young's modulus of rubber is closest to ______.
The force required to stretch a wire of cross section 1 cm2 to double its length will be ______.
(Given Young's modulus of the wire = 2 × 1011 N/m2)
