Advertisements
Advertisements
Question
A 14.5 kg mass, fastened to the end of a steel wire of unstretched length 1.0 m, is whirled in a vertical circle with an angular velocity of 2 rev/s at the bottom of the circle. The cross-sectional area of the wire is 0.065 cm2. Calculate the elongation of the wire when the mass is at the lowest point of its path.
Solution 1
Mass, m = 14.5 kg
Length of the steel wire, l = 1.0 m
Angular velocity, ω = 2 rev/s = 2 × 2π rad/s = 12.56 rad/s
Cross-sectional area of the wire, a = 0.065 cm2 = 0.065 × 10-4 m2
Let Δl be the elongation of the wire when the mass is at the lowest point of its path.
When the mass is placed at the position of the vertical circle, the total force on the mass is:
F = mg + mlω2
= 14.5 × 9.8 + 14.5 × 1 × (12.56)2
= 2429.53 N
Young's modulus = Stress/Strain
`Y = (F/A)/trianglel = F/A l/trianglel`
`:.trianglel = (Fl)/(AY)`
Young’s modulus for steel = 2 × 1011 Pa
`trianglel = (2429.53xx1)/(0.065xx10^(-4)xx2xx10^11)`
`=>trianglel = 1.87 xx 10^(-3) m`
Hence, the elongation of the wire is 1.87 × 10–3 m.
Solution 2
Here, m = 14.5 kg; l = r = 1 m; v = 2 rps; A = 0.065 x 10-4 m2 Total pulling force on mass, when it is at the lowest position of the vertical circle is F = mg + mr w2 = mg + mr 4,π2 v2
`=14.5 xx 9.8 + 14.5 xx 1 xx 4 xx (22/7)^2 xx 2^2`
=142.1 + 2291.6 = 2433.9 N
`Y = F/A xx l/(trianglel)`
or `trianglel = (Fl)/(AY)= (2433.7xx1)/(0.065 xx 10^(-4)xx(2xx10^11)) = 1.87 xx 10^(-3) m = 1.87 m`
APPEARS IN
RELATED QUESTIONS
A steel wire of length 4.7 m and cross-sectional area 3.0 × 10–5 m2 stretches by the same amount as a copper wire of length 3.5 m and cross-sectional area of 4.0 × 10–5 m2 under a given load. What is the ratio of Young’s modulus of steel to that of copper?
Four identical hollow cylindrical columns of mild steel support a big structure of mass 50,000 kg. The inner and outer radii of each column are 30 cm and 60 cm respectively. Assuming the load distribution to be uniform, calculate the compressional strain of each column.
A student plots a graph from his reading on the determination of Young modulus of a metal wire but forgets to put the labels. the quantities on X and Y-axes may be respectively
(a) weight hung and length increased
(b) stress applied and length increased
(c) stress applied and strain developed
(d) length increased and the weight hung.
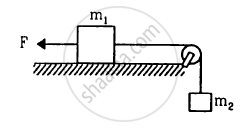
Consider the situation shown in figure. The force F is equal to the m2 g/2. If the area of cross section of the string is A and its Young modulus Y, find the strain developed in it. The string is light and there is no friction anywhere.
The temperature of a wire is doubled. The Young’s modulus of elasticity ______.
Identical springs of steel and copper are equally stretched. On which, more work will have to be done?
What is the Young’s modulus for a perfect rigid body ?
A steel rod (Y = 2.0 × 1011 Nm–2; and α = 10–50 C–1) of length 1 m and area of cross-section 1 cm2 is heated from 0°C to 200°C, without being allowed to extend or bend. What is the tension produced in the rod?
A truck is pulling a car out of a ditch by means of a steel cable that is 9.1 m long and has a radius of 5 mm. When the car just begins to move, the tension in the cable is 800 N. How much has the cable stretched? (Young’s modulus for steel is 2 × 1011 Nm–2.)
A steel wire of mass µ per unit length with a circular cross section has a radius of 0.1 cm. The wire is of length 10 m when measured lying horizontal, and hangs from a hook on the wall. A mass of 25 kg is hung from the free end of the wire. Assuming the wire to be uniform and lateral strains << longitudinal strains, find the extension in the length of the wire. The density of steel is 7860 kg m–3 (Young’s modules Y = 2 × 1011 Nm–2).
