Advertisements
Advertisements
प्रश्न
A copper wire of cross-sectional area 0.01 cm2 is under a tension of 20N. Find the decrease in the cross-sectional area. Young modulus of copper = 1.1 × 1011 N m−2 and Poisson ratio = 0.32.
`["Hint" : (Delta"A")/"A"=2(Delta"r")/"r"]`
उत्तर
Given:
Cross-sectional area of copper wire A = 0.01 cm2 = 10−6 m2
Applied tension T = 20 N
Young modulus of copper Y = 1.1 × 1011 N/m2
Poisson ratio σ = 0.32
We know that: \[Y = \frac{FL}{A ∆ L}\]
\[\Rightarrow \frac{∆ L}{L} = \frac{F}{AY}\]
\[ = \frac{20}{{10}^{- 6} \times 1 . 1 \times {10}^{11}} = 18 . 18 \times {10}^{- 5} \]
\[\text{ Poisson's ratio }, \sigma = \frac{\frac{∆ d}{d}}{\frac{∆ L}{L}} = 0 . 32\]
\[\text{ Where d is the transverse length }\]
\[\text{ So }, \frac{∆ d}{d} = \left( 0 . 32 \right) \times \frac{∆ L}{L}\]
\[ = 0 . 32 \times \left( 18 . 18 \right) \times {10}^{- 5} = 5 . 81 \times {10}^{- 5} \]
\[\text{ Again }, \frac{∆ A}{A} = \frac{2 ∆ r}{r} = \frac{2 ∆ d}{d}\]
\[ \Rightarrow ∆ A = \frac{2 ∆ d}{d}A\]
\[ \Rightarrow ∆ A = 2 \times \left( 5 . 8 \times {10}^{- 5} \right) \times \left( 0 . 01 \right)\]
\[ = 1 . 164 \times {10}^{- 6} {\text{ cm }}^2\]
Hence, the required decrease in the cross -sectional area is \[1 . 164 \times {10}^{- 6} {\text{ cm } }^2\]
APPEARS IN
संबंधित प्रश्न
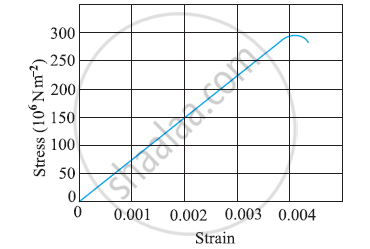
The figure shows the strain-stress curve for a given material. What are (a) Young’s modulus and (b) approximate yield strength for this material?
The stress-strain graphs for materials A and B are shown in Figure
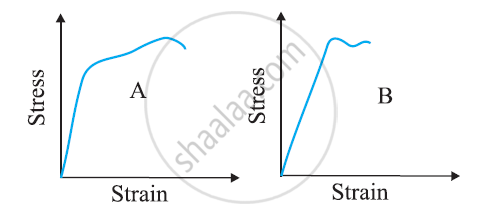
The graphs are drawn to the same scale.
(a) Which of the materials has the greater Young’s modulus?
(b) Which of the two is the stronger material?
Read the following statements below carefully and state, with reasons, if it is true or false
The Young’s modulus of rubber is greater than that of steel;
Four identical hollow cylindrical columns of mild steel support a big structure of mass 50,000 kg. The inner and outer radii of each column are 30 cm and 60 cm respectively. Assuming the load distribution to be uniform, calculate the compressional strain of each column.
Two wires A and B are made of same material. The wire A has a length l and diameter rwhile the wire B has a length 2l and diameter r/2. If the two wires are stretched by the same force, the elongation in A divided by the elongation in B is
A wire elongates by 1.0 mm when a load W is hung from it. If this wire goes over a a pulley and two weights W each are hung at the two ends, he elongation of he wire will be
A student plots a graph from his reading on the determination of Young modulus of a metal wire but forgets to put the labels. the quantities on X and Y-axes may be respectively
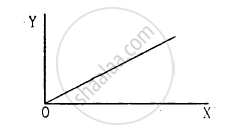
(a) weight hung and length increased
(b) stress applied and length increased
(c) stress applied and strain developed
(d) length increased and the weight hung.
Young's modulus of a perfectly rigid body is ______.
The temperature of a wire is doubled. The Young’s modulus of elasticity ______.
The Young’s modulus for steel is much more than that for rubber. For the same longitudinal strain, which one will have greater tensile stress?
Identical springs of steel and copper are equally stretched. On which, more work will have to be done?
A truck is pulling a car out of a ditch by means of a steel cable that is 9.1 m long and has a radius of 5 mm. When the car just begins to move, the tension in the cable is 800 N. How much has the cable stretched? (Young’s modulus for steel is 2 × 1011 Nm–2.)
If the yield strength of steel is 2.5 × 108 Nm–2, what is the maximum weight that can be hung at the lower end of the wire?
A steel rod of length 2l, cross sectional area A and mass M is set rotating in a horizontal plane about an axis passing through the centre. If Y is the Young’s modulus for steel, find the extension in the length of the rod. (Assume the rod is uniform.)
If Y, K and η are the values of Young's modulus, bulk modulus and modulus of rigidity of any material respectively. Choose the correct relation for these parameters.
A boy's catapult is made of rubber cord which is 42 cm long, with a 6 mm diameter of cross-section and negligible mass. The boy keeps a stone weighing 0.02 kg on it and stretches the cord by 20 cm by applying a constant force. When released, the stone flies off with a velocity of 20 ms-1. Neglect the change in the area of the cross-section of the cord while stretched. Young's modulus of rubber is closest to ______.
A uniform metal rod of 2 mm2 cross section is heated from 0°C to 20°C. The coefficient of linear expansion of the rod is 12 × 10-6/°C, it's Young's modulus is 1011 N/m2. The energy stored per unit volume of the rod is ______.
The force required to stretch a wire of cross section 1 cm2 to double its length will be ______.
(Given Young's modulus of the wire = 2 × 1011 N/m2)
