Advertisements
Advertisements
Question
A current of 10 A is established in a long wire along the positive z-axis. Find the magnetic field \[\vec{B}\] at the point (1 m, 0, 0).
Solution
Given:
Magnitude of current, I = 10 A
Separation of the point from the wire, d = 1 m
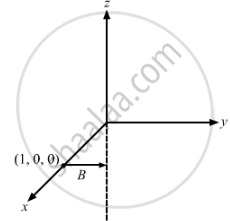
The magnetic field \[\vec{B}\] at point (1 m, 0, 0) is given by
\[B = \frac{\mu_0 i}{2\pi d} = \frac{4\pi \times {10}^{- 7} \times 10}{2\pi \times 1}\]
\[ \Rightarrow B = 2 \times {10}^{- 6} T\]
(Along the +ve y-axis by the right-hand thumb rule)
APPEARS IN
RELATED QUESTIONS
How does one understand this motional emf by invoking the Lorentz force acting on the free charge carriers of the conductor? Explain.
What is the magnitude of magnetic force per unit length on a wire carrying a current of 8 A and making an angle of 30° with the direction of a uniform magnetic field of 0.15 T?
A long, straight wire of radius R carries a current distributed uniformly over its cross section. T he magnitude of the magnetic field is
(a) maximum at the axis of the wire
(b) minimum at the axis of the wire
(c) maximum at the surface of the wire
(d) minimum at the surface of the wire.
A copper wire of diameter 1.6 mm carries a current of 20 A. Find the maximum magnitude of the magnetic field `vecB` due to this current.
A transmission wire carries a current of 100 A. What would be the magnetic field B at a point on the road if the wire is 8 m above the road?
A long, straight wire carrying a current of 1.0 A is placed horizontally in a uniform magnetic field B = 1.0 × 10−5 T pointing vertically upward figure. Find the magnitude of the resultant magnetic field at the points P and Q, both situated at a distance of 2.0 cm from the wire in the same horizontal plane.

A straight wire of length l can slide on two parallel plastic rails kept in a horizontal plane with a separation d. The coefficient of friction between the wire and the rails is µ. If the wire carries a current i, what minimum magnetic field should exist in the space in order to slide the wire on the rails?
Figure shows two parallel wires separated by a distance of 4.0 cm and carrying equal currents of 10 A along opposite directions. Find the magnitude of the magnetic field B at the points A1, A2, A3.

A long, straight wire carries a current i. Let B1 be the magnetic field at a point P at a distance d from the wire. Consider a section of length l of this wire such that the point P lies on a perpendicular bisector of the section B2 be the magnetic field at this point due to this second only. Find the value of d/l so that B2 differs from B1 by 1%.
A straight, how wire carries a current of 20 A. Another wire carrying equal current is placed parallel to it. If the force acting on a length of 10 cm of the second wire is 2.0 × 10−5 N, what is the separation between them?
If a current I is flowing in a straight wire parallel to x-axis and magnetic field is there in the y-axis then, ______.
The nature of parallel and anti-parallel currents are ______.
Equal currents are passing through two very long and straight parallel wires in the same direction. They will ______
Beams of electrons and protons move parallel to each other in the same direction. They ______.
Two long parallel wires kept 2 m apart carry 3A current each, in the same direction. The force per unit length on one wire due to the other is ______.
The figure below are two long, parallel wires carrying current in the same direction such that I1 < I2.

- In which direction will wire I1 move?
- If the direction of the current I2 is reversed, in which direction will the wire I1 move now?
