Advertisements
Advertisements
Question
A line segment is of length 10 units. If the coordinates of its one end are (2, −3) and the abscissa of the other end is 10, then its ordinate is
Options
9, 6
3, −9
−3, 9
9, −6
Solution
It is given that distance between P (2,−3) and Q(10 , y) is 10.
In general, the distance between A(x1 , y1) and B(x2 , y2 ) is given by,
`AB^2 = (x_2 - x_1 )^2 + (y_2 - y_1 )^2`
So,
`10^2 = (10 - 2)^2 + (y + 3)^2`
On further simplification,
`(y + 3 )^2 = 36`
`y = -3+-6`
`= -9 , 3`
We will neglect the negative value. So,
` y= -9 , 3`
APPEARS IN
RELATED QUESTIONS
(Street Plan): A city has two main roads which cross each other at the centre of the city. These two roads are along the North-South direction and East-West direction.
All the other streets of the city run parallel to these roads and are 200 m apart. There are 5 streets in each direction. Using 1cm = 200 m, draw a model of the city on your notebook. Represent the roads/streets by single lines.
There are many cross- streets in your model. A particular cross-street is made by two streets, one running in the North - South direction and another in the East - West direction. Each cross street is referred to in the following manner : If the 2nd street running in the North - South direction and 5th in the East - West direction meet at some crossing, then we will call this cross-street (2, 5). Using this convention, find:
- how many cross - streets can be referred to as (4, 3).
- how many cross - streets can be referred to as (3, 4).
On which axis do the following points lie?
Q(0, -2)
Prove that the points (0, 0), (5, 5) and (-5, 5) are the vertices of a right isosceles triangle.
Find the points of trisection of the line segment joining the points:
(2, -2) and (-7, 4).
Prove that the points A(-4,-1), B(-2, 4), C(4, 0) and D(2, 3) are the vertices of a rectangle.
Find the points on the x-axis, each of which is at a distance of 10 units from the point A(11, –8).
Show that the points A(3,0), B(4,5), C(-1,4) and D(-2,-1) are the vertices of a rhombus. Find its area.
The base QR of a n equilateral triangle PQR lies on x-axis. The coordinates of the point Q are (-4, 0) and origin is the midpoint of the base. Find the coordinates of the points P and R.
Find the ratio in which the point (-1, y) lying on the line segment joining points A(-3, 10) and (6, -8) divides it. Also, find the value of y.
Find the coordinates of circumcentre and radius of circumcircle of ∆ABC if A(7, 1), B(3, 5) and C(2, 0) are given.
The area of the triangle formed by the points P (0, 1), Q (0, 5) and R (3, 4) is
ABCD is a parallelogram with vertices \[A ( x_1 , y_1 ), B \left( x_2 , y_2 \right), C ( x_3 , y_3 )\] . Find the coordinates of the fourth vertex D in terms of \[x_1 , x_2 , x_3 , y_1 , y_2 \text{ and } y_3\]
Write the coordinates of the point dividing line segment joining points (2, 3) and (3, 4) internally in the ratio 1 : 5.
Write the ratio in which the line segment doining the points A (3, −6), and B (5, 3) is divided by X-axis.
Find the distance between the points \[\left( - \frac{8}{5}, 2 \right)\] and \[\left( \frac{2}{5}, 2 \right)\] .
If P is a point on x-axis such that its distance from the origin is 3 units, then the coordinates of a point Q on OY such that OP = OQ, are
If A(x, 2), B(−3, −4) and C(7, −5) are collinear, then the value of x is
Seg AB is parallel to X-axis and coordinates of the point A are (1, 3), then the coordinates of the point B can be ______.
A tiling or tessellation of a flat surface is the covering of a plane using one or more geometric shapes, called tiles, with no overlaps and no gaps. Historically, tessellations were used in ancient Rome and in Islamic art. You may find tessellation patterns on floors, walls, paintings etc. Shown below is a tiled floor in the archaeological Museum of Seville, made using squares, triangles and hexagons.

A craftsman thought of making a floor pattern after being inspired by the above design. To ensure accuracy in his work, he made the pattern on the Cartesian plane. He used regular octagons, squares and triangles for his floor tessellation pattern
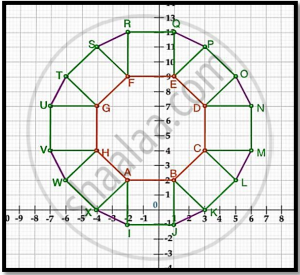
Use the above figure to answer the questions that follow:
- What is the length of the line segment joining points B and F?
- The centre ‘Z’ of the figure will be the point of intersection of the diagonals of quadrilateral WXOP. Then what are the coordinates of Z?
- What are the coordinates of the point on y-axis equidistant from A and G?
OR
What is the area of Trapezium AFGH?
