Advertisements
Advertisements
Question
A mass of 5 kg is moving along a circular path of radius 1 m. If the mass moves with 300 revolutions per minute, its kinetic energy would be ______.
Options
250 π2
100 π2
5 π2
0
Solution
A mass of 5 kg is moving along a circular path of radius 1 m. If the mass moves with 300 revolutions per minute, its kinetic energy would be `underline(250 π^2)`.
Explanation:
Given, mass = m = 5 kg
Radius = 1 m = R
Revolution per minute ω = 300 rev/min
= (300 × 2π) rad/min
= (300 × 2 × 3.14) rad/60 s
= `(300 xx 2 xx 3.14)/60` rad/s
= 10 × rad/s
⇒ Linear speed = v = ωR
= `((300 xx 2π)/60) (1m)`
= 10π m/s
KE = `1/2 mv^2`
= `1/2 xx 5 xx (10π)^2`
= `100π^2 xx 5 xx 1/2`
= 250 π2 J
APPEARS IN
RELATED QUESTIONS
A person trying to lose weight (dieter) lifts a 10 kg mass, one thousand times, to a height of 0.5 m each time. Assume that the potential energy lost each time she lowers the mass is dissipated.
- How much work does she do against the gravitational force?
- Fat supplies 3.8 x 107J of energy per kilogram which is converted to mechanical energy with a 20% efficiency rate. How much fat will the dieter use up?
A heavy stone is thrown from a cliff of height h with a speed v. The stoen will hit the ground with maximum speed if it is thrown
You lift a suitcase from the floor and keep it on a table. The work done by you on the suitcase does not depend on
(a) the path taken by the suitcase
(b) the time taken by you in doing so
(c) the weight of the suitcase
(d) your weight
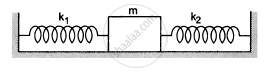
A block of mass m is attached to two unstretched springs of spring constants k1 and k2 as shown in the following figure. The block is displaced towards the right through a distance x and is released. Find the speed of the block as it passes through the mean position shown.
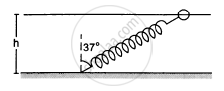
One end of a spring of natural length h and spring constant k is fixed at the ground and the other is fitted with a smooth ring of mass m which is allowed to slide on a horizontal rod fixed at a height h (following figure). Initially, the spring makes an angle of 37° with the vertical when the system is released from rest. Find the speed of the ring when the spring becomes vertical.
A spring of negligible mass and force constant 5 Nm–1 is compressed by a distance x = 5 cm. A block of mass 200 g is free to leave the end of the spring. If the system is released, what will be the speed of the block when it leaves the spring?
A particle is released from height S from the surface of the Earth. At a certain height, its kinetic energy is three times its potential energy. The height from the surface of the earth and the speed of the particle at that instant are respectively ______.
Why is electrical power required at all when the elevator is descending? Why should there be a limit on the number of passengers in this case?
A body falls towards earth in air. Will its total mechanical energy be conserved during the fall? Justify.
A single conservative force acts on a body of mass 1 kg that moves along the x-axis. The potential energy U(x) is given by U (x) = 20 + (x - 2)2, where x is in meters. At x = 5.0 m the particle has a kinetic energy of 20 J, then the maximum kinetic energy of body is ______ J.
