Advertisements
Advertisements
Question
A monkey of mass 40 kg climbs on a rope in given Figure which can stand a maximum tension of 600 N. In which of the following cases will the rope break: the monkey
(a) climbs up with an acceleration of 6 m s–2
(b) climbs down with an acceleration of 4 m s–2
(c) climbs up with a uniform speed of 5 m s–1
(d) falls down the rope nearly freely under gravity?
(Ignore the mass of the rope).
Advertisements
Solution 1
Case (a)
Mass of the monkey, m = 40 kg
Acceleration due to gravity, g = 10 m/s
Maximum tension that the rope can bear, Tmax = 600 N
Acceleration of the monkey, a = 6 m/s2 upward
Using Newton’s second law of motion, we can write the equation of motion as:
T – mg = ma
∴T = m(g + a)
= 40 (10 + 6)
= 640 N
Since T > Tmax, the rope will break in this case.
Case (b)
Acceleration of the monkey, a = 4 m/s2 downward
Using Newton’s second law of motion, we can write the equation of motion as:
mg – T = ma
∴T = m (g – a)
= 40(10 – 4)
= 240 N
Since T < Tmax, the rope will not break in this case.
Case (c)
The monkey is climbing with a uniform speed of 5 m/s. Therefore, its acceleration is zero, i.e., a = 0.
Using Newton’s second law of motion, we can write the equation of motion as:
T – mg = ma
T – mg = 0
∴T = mg
= 40 × 10
= 400 N
Since T < Tmax, the rope will not break in this case.
Case (d)
When the monkey falls freely under gravity, its will acceleration become equal to the acceleration due to gravity, i.e., a = g
Using Newton’s second law of motion, we can write the equation of motion as:
mg – T = mg
∴T = m(g – g) = 0
Since T < Tmax, the rope will not break in this case.
Solution 2
(a) When the monkey climbs up with an acceleration a, then T – mg = ma where T represents the tension in figure.
:. T = mg + ma = m (g +a)
or T = 40 kg(10 + 6) ms^(-2) = 640 N
But the rope can withstand a maximum tension of 600 N. So the rope will break
b) When the monkey is climbing down with an acceleration, then
mg - T =ma (Figure b)
=> T = mg - ma = m(g -a)
or T = 40 kg x (10 - 4) `ms^2` = 240 N
(c) When the monkey climbs up with uniform speed, then T mg = 40 kg x 10 ms-2 = 400 N The rope will hot break.
(d) When the monkey is falling freely, it would be a state of weightlessness. So, tension will be zero and the rope will not break.
RELATED QUESTIONS
A constant retarding force of 50 N is applied to a body of mass 20 kg moving initially with a speed of 15 ms–1. How long does the body take to stop?
A man of mass 70 kg stands on a weighing scale in a lift which is moving
- upwards with a uniform speed of 10 m s-1
- downwards with a uniform acceleration of 5 m s–2
- upwards with a uniform acceleration of 5 m s–2. What would be the readings on the scale in each case?
- What would be the reading if the lift mechanism failed and it hurtled down freely under gravity?
Two masses 8 kg and 12 kg are connected at the two ends of a light, inextensible string that goes over a frictionless pulley. Find the acceleration of the masses, and the tension in the string when the masses are released.
An object is placed far away from all the objects that can exert force on it. A frame of reference is constructed by taking the origin and axes fixed in this object. Will the frame be necessarily inertial?
The figure shows the displacement of a particle going along the X-axis as a function of time. The force acting on the particle is zero in the region
(a) AB
(b) BC
(c) CD
(d) DE
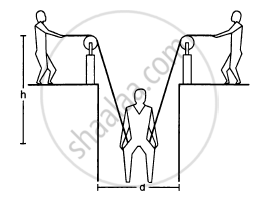
Find the mass M of the hanging block in the following figure that will prevent the smaller block from slipping over the triangular block. All the surfaces are frictionless and the strings and the pulleys are light.
Show that the rate of change of momentum = mass × acceleration. Under what condition does this relation hold?
A force acts for 10 s on a stationary body of mass 100 kg, after which the force ceases to act. The body moves through a distance of 100 m in the next 5 s. Calculate: The velocity acquired by the body.
A pebble is thrown vertically upwards with a speed of 20 m s-1. How high will it be after 2 s? (Take g = 10 m s-2)
A pebble is dropped freely in a well from its top. It takes 20 s for the pebble to reach the water surface in the well. Taking g = 10 m s-2 and speed of sound = 330 m s-1. Find : The depth of water surface
Calculate the velocity of a body of mass 0.5 kg, when it has a linear momentum of 5 Ns.
Multiple Choice Question. Select the correct option.
Which of the following are vector quantities?
A stone is dropped from a cliff 98 m high.
What will be its speed when it strikes the ground?
A stone is thrown vertically upward with a velocity of 9.8 m/s. When will it reach the ground?
In the previous problem (5.3), the magnitude of the momentum transferred during the hit is ______.
A hockey player is moving northward and suddenly turns westward with the same speed to avoid an opponent. The force that acts on the player is ______.
A body of mass 2 kg travels according to the law x(t) = pt + qt2 + rt3 where p = 3 ms−1, q = 4 ms−2 and r = 5 ms−3. The force acting on the body at t = 2 seconds is ______.
The motion of a particle of mass m is given by x = 0 for t < 0 s, x(t) = A sin 4 pt for 0 < t < (1/4) s (A > o), and x = 0 for t > (1/4) s. Which of the following statements is true?
- The force at t = (1/8) s on the particle is – 16π2 Am.
- The particle is acted upon by on impulse of magnitude 4π2 A m at t = 0 s and t = (1/4) s.
- The particle is not acted upon by any force.
- The particle is not acted upon by a constant force.
- There is no impulse acting on the particle.
A woman throws an object of mass 500 g with a speed of 25 ms1.
- What is the impulse imparted to the object?
- If the object hits a wall and rebounds with half the original speed, what is the change in momentum of the object?
According to Newton's Second Law of Motion, what quantity is directly proportional to the applied force?
