Advertisements
Advertisements
Question
A rectangular path of 60 m length and 3 m width is covered by square tiles of side 25 cm. How many tiles will there be in one row along its width? How many such rows will be there? Find the number of tiles used to make this path?
Solution
Given, Length of path = 60 m
And width of path = 30 m
Side of square tile = 25 cm = `25/100` m = 0.25 m .....[∵ 1 m = 100 cm]
Diagram of path is shown below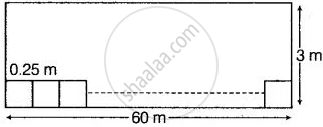
Number of tiles in one row along width = `"Width"/"Side of one tile" = 3/0.25 = (3 xx 100)/25` = 12
Number of rows = `"Length"/"Side of one tile" = 60/0.25 = 60/25 xx 100` = 240
Also, number of tiles = Number of tiles in one row × Number of rows = 12 × 240 = 2880
APPEARS IN
RELATED QUESTIONS
The length of a rectangle is 16 cm and its perimeter is equal to the perimeter of a square with side 12.5 cm. Find the area of the rectangle.
Find the perimeter of a rectangle whose:
length = 40 cm and breadth = 35 cm
Each side of a square plot of land is 55 m. Find the cost of fencing the plot at the rate of ₹32 per meter.
Each side of a square field is 70 cm. How much distance will a boy walk in order to make?
(i) one complete round of this field?
(ii) 8 complete rounds of this field?
Mohit makes 8 full rounds of a rectangular field with length = 120 m and breadth = 75 m.
John makes 10 full rounds of a square field with each side 100 in. Find who covers larger distance and by how much?
Find the perimeter of an equilateral triangle of side 9.8 cm.
A rectangle with length = 18 cm and breadth = 12 cm has same perimeter as that of a regular pentagon. Find the side of the pentagon.
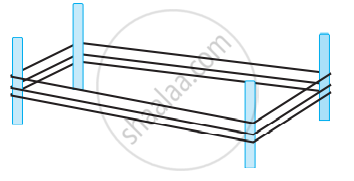
A farmer has a rectangular field of length and breadth 240 m and 180 m respectively. He wants to fence it with 3 rounds of rope as shown in the figure. What is the total length of rope he must use?
Rahim and Peter go for a morning walk, Rahim walks around a square path of side 50 m and Peter walks around a rectangular path with length 40 m and breadth 30 m. If both of them walk 2 rounds each, who covers more distance and by how much?
In the given figure, perimeter of (ii) is greater than that of (i), but its area is smaller than that of (i).
 |
 |
| (i) | (ii) |
