Advertisements
Advertisements
Question
A wave moving in a gas
Options
must be longitudinal
may be longitudinal
must transverse
may be transverse.
Solution
must be longitudinal
Because particles in a gas are far apart, only longitudinal wave can travel through it.
APPEARS IN
RELATED QUESTIONS
When longitudinal wave is incident at the boundary of denser medium, then............................
- compression reflects as a compression.
- compression reflects as a rarefaction.
- rarefaction reflects as a compression.
- longitudinal wave reflects as transverse wave.
A wire of density ‘ρ’ and Young’s modulus ‘Y’ is stretched between two rigid supports separated by a distance ‘L’ under tension ‘T’. Derive an expression for its frequency in fundamental mode. Hence show that `n=1/(2L)sqrt((Yl)/(rhoL))` where symbols have their usual meanings
Explain why (or how) The shape of a pulse gets distorted during propagation in a dispersive medium.
Explain the reflection of transverse and longitudinal waves from a denser medium and a rared medium.
You are walking along a seashore and a mild wind is blowing. Is the motion of air a wave motion?
Mark out the correct options.
A particle on a stretched string supporting a travelling wave, takes 5⋅0 ms to move from its mean position to the extreme position. The distance between two consecutive particles, which are at their mean positions, is 2⋅0 cm. Find the frequency, the wavelength and the wave speed.
A vertical rod is hit at one end. What kind of wave propagates in the rod if (a) the hit is made vertically (b) the hit is made horizontally?
A transverse wave described by \[y = \left( 0 \cdot 02 m \right) \sin \left( 1 \cdot 0 m^{- 1} \right) x + \left( 30 s^{- 1} \right)t\] propagates on a stretched string having a linear mass density of \[1 \cdot 2 \times {10}^{- 4} kg m^{- 1}\] the tension in the string.
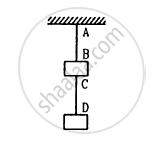
Two blocks each having a mass of 3⋅2 kg are connected by a wire CD and the system is suspended from the ceiling by another wire AB (See following figure). The linear mass density of the wire AB is 10 g m−1 and that of CD is 8 g m−1. Find the speed of a transverse wave pulse produced in AB and CD.
An organ pipe, open at both ends, contains
If the speed of a transverse wave on a stretched string of length 1 m is 60 m−1, what is the fundamental frequency of vibration?
A steel wire of mass 4⋅0 g and length 80 cm is fixed at the two ends. The tension in the wire is 50 N. Find the frequency and wavelength of the fourth harmonic of the fundamental.
Three resonant frequencies of a string are 90, 150 and 210 Hz. (a) Find the highest possible fundamental frequency of vibration of this string. (b) Which harmonics of the fundamental are the given frequencies? (c) Which overtones are these frequencies? (d) If the length of the string is 80 cm, what would be the speed of a transverse wave on this string?
The equation of a standing wave, produced on a string fixed at both ends, is
\[y = \left( 0 \cdot 4 cm \right) \sin \left[ \left( 0 \cdot 314 {cm}^{- 1} \right) x \right] \cos \left[ \left( 600\pi s^{- 1} \right) t \right]\]
What could be the smallest length of the string?
Given below are some functions of x and t to represent the displacement (transverse or longitudinal) of an elastic wave. State which of these represent (i) a traveling wave, (ii) a stationary wave or (iii) none at all:
y = 2 cos (3x) sin (10t)
Given below are some functions of x and t to represent the displacement (transverse or longitudinal) of an elastic wave. State which of these represent (i) a traveling wave, (ii) a stationary wave or (iii) none at all:
`"y" = 2sqrt(x - "vt")`
Given below are some functions of x and t to represent the displacement (transverse or longitudinal) of an elastic wave. State which of these represent (i) a traveling wave, (ii) a stationary wave or (iii) none at all:
y = 3 sin (5x – 0.5t) + 4 cos (5x – 0.5t)
