Advertisements
Advertisements
Question
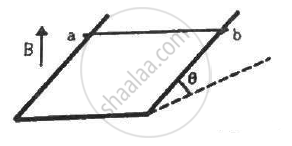
A wire ab of length l, mass m and resistance R slides on a smooth, thick pair of metallic rails joined at the bottom as shown in figure. The plane of the rails makes an angle θ with the horizontal. A vertical magnetic field B exists in the region. If the wire slides on the rails at a constant speed v, show that \[B = \sqrt{\frac{mg R sin\theta}{v l^2 \cos^2 \theta}}\]
Solution
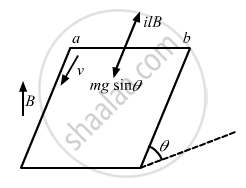
Component of weight along its motion, F' = mgsinθ
The emf induced in the rod due to its motion is given by
e = Bl'v'
Here,
l' = Component of the length of the rod perpendicular to the magnetic field
v' = Component of the velocity of the rod perpendicular to the magnetic field
\[i = \frac{B \times l \times v cos\theta}{R}\]
\[\left| \overrightarrow{F} \right| = i\left| \overrightarrow{l} \times \overrightarrow{B} \right| = ilB\sin(90 - \theta)\]
\[F = ilB = \frac{Blv \cos\theta}{R} \times l \times B\cos\theta\]
\[F = \frac{B^2 l^2 v \cos^2 \theta}{R}\]
The direction of force F is opposite to F.'
Because the rod is moving with a constant velocity, the net force on it is zero.
Thus,
F - F' = 0
F = F'
or
\[\frac{B^2 l^2 v \cos^2 \theta}{R} = mg\sin\theta\]
\[\therefore B = \sqrt{\frac{Rmg\sin\theta}{l^2 v \cos^2 \theta}}\]
APPEARS IN
RELATED QUESTIONS
A point charge q moving with speed v enters a uniform magnetic field B that is acting into the plane of the paper as shown. What is the path followed by the charge q and in which plane does it move?
If an electric field \[\vec{E}\] is also applied such that the particle continues moving along the original straight line path, what should be the magnitude and direction of the electric field \[\vec{E}\] ?

The motion of copper plate is damped when it is allowed to oscillate between the two poles of a magnet. What is the cause of this damping?
A moving charge produces
Consider the situation shown in figure. The wires P1Q1 and P2Q2 are made to slide on the rails with the same speed 5 cm s−1. Suppose the 19 Ω resistor is disconnected. Find the current through P2Q2 if (a) both the wires move towards right and (b) if P1Q1 moves towards left but P2Q2 moves towards right.

A magnetic field that varies in magnitude from point to point but has a constant direction (east to west) is set up in a chamber. A charged particle enters the chamber and travels undeflected along a straight path with constant speed. What can you say about the initial velocity of the particle?
A charged particle moves through a magnetic field perpendicular to its direction. Then ______.
If an electron is moving with velocity `vecnu` produces a magnetic field `vec"B"`, then ______.
A charged particle moving in a magnetic field experiences a resultant force ______
A thin strip 10 cm long is on a U-shaped wire of negligible resistance and it is connected to a spring of spring constant 0.5 Nm-1. The assembly is kept in a uniform magnetic field of 0.1 T. If the strip is pulled from its equilibrium position and released, the number of oscillations it performs before its amplitude decreases by a factor of e is N. If the mass of the strip is 50 grams, its resistance is 10 Ω, and air drag is negligible, N will be close to ______.

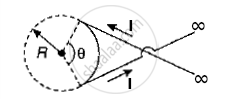
A wire carrying current i has the configuration shown in figure. For the magnetic field to be zero at the centre of the circle, θ must be:
A conductor ABOCD moves along its bisector with a velocity 1 m/s through a perpendicular magnetic field of 1 wb/m2, as shown in figure. If all the four sides are 1 m length each, then the induced emf between A and Din approx is ______V.

Protons and singly ionized atoms of U235 and U238 are passed in turn (which means one after the other and not at the same time) through a velocity selector and then enter a uniform magnetic field. The protons describe semicircles of radius 10 mm. The separation between the ions of U235 and U238 after describing the semicircle is given by ______.
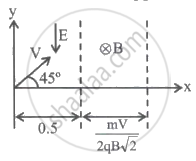
A charged particle of charge q and mass m is projected in a region that contains an electric and magnetic field as shown in the figure with velocity V at an angle of 45° with x-direction. If V = `sqrt((qE)/m)`, then net deviation in particle motion will be (neglect the effect of gravity) in a clockwise direction approx ______ °.