Advertisements
Advertisements
Question
|
Case Study Trigonometry in the form of triangulation forms the basis of navigation, whether it is by land, sea or air. GPS a radio navigation system helps to locate our position on earth with the help of satellites. |
- Make a labelled figure on the basis of the given information and calculate the distance of the boat from the foot of the observation tower.
- After 10 minutes, the guard observed that the boat was approaching the tower and its distance from tower is reduced by 240(`sqrt(3)` - 1) m. He immediately raised the alarm. What was the new angle of depression of the boat from the top of the observation tower?
Advertisements
Solution
i.
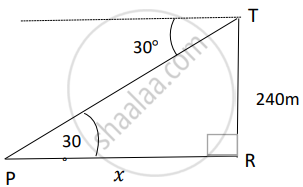
In ∆PTR, tan 30° = `240/x` ⇒ x = `240sqrt(3)` m
ii. Distance of boat from tower = `240sqrt(3) - 240(sqrt(3) - 1)` = 240 m
Let the angle of depression = θ
tan θ = `240/240` = 1 ⇒ θ = 45°
APPEARS IN
RELATED QUESTIONS
If the point (x, y) is equidistant from the points (a + b, b – a) and (a – b, a + b), prove that bx = ay.
Find the values of y for which the distance between the points P (2, -3) and Q (10, y) is 10 units.
Find all possible values of x for which the distance between the points
A(x,-1) and B(5,3) is 5 units.
Find the distances between the following point.
P(–6, –3), Q(–1, 9)
Find the distance of the following point from the origin :
(0 , 11)
Find the coordinate of O , the centre of a circle passing through A (8 , 12) , B (11 , 3), and C (0 , 14). Also , find its radius.
The points A (3, 0), B (a, -2) and C (4, -1) are the vertices of triangle ABC right angled at vertex A. Find the value of a.
Give the relation that must exist between x and y so that (x, y) is equidistant from (6, -1) and (2, 3).
Show that A(1, 2), (1, 6), C(1 + 2 `sqrt(3)`, 4) are vertices of a equilateral triangle
Case Study -2
A hockey field is the playing surface for the game of hockey. Historically, the game was played on natural turf (grass) but nowadays it is predominantly played on an artificial turf.
It is rectangular in shape - 100 yards by 60 yards. Goals consist of two upright posts placed equidistant from the centre of the backline, joined at the top by a horizontal crossbar. The inner edges of the posts must be 3.66 metres (4 yards) apart, and the lower edge of the crossbar must be 2.14 metres (7 feet) above the ground.
Each team plays with 11 players on the field during the game including the goalie. Positions you might play include -
- Forward: As shown by players A, B, C and D.
- Midfielders: As shown by players E, F and G.
- Fullbacks: As shown by players H, I and J.
- Goalie: As shown by player K.
Using the picture of a hockey field below, answer the questions that follow:
The point on x axis equidistant from I and E is ______.
