Advertisements
Advertisements
Question
Construct an isosceles ∆ PQR such that PQ = PR = 6.5 cm and ∠PQR = 75°. Using a ruler and compasses only constructs a circumcircle to this triangle.
Solution
Steps of Construction :
(i) Draw a line segment PQ = 6.5 cm
(ii) At Q, draw a ray making an angle of 75°.
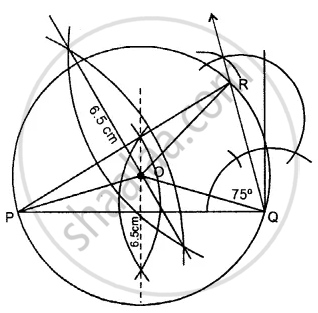
(iii) Through P, with a radius of 6.5 cm, draw an arc which intersects the angle ray at R.
(iv) Join PR,
Δ PQR is the required triangle.
(v) Draw the perpendicular bisectors of sides PQ and PR intersecting each other at O.
(vi) Join OP, OQ and OR.
(vii) With centre O and radius equal to OP or OQ or OR draw a circle which passes through P, Q and R. This is the required circumcircle of Δ PQR
APPEARS IN
RELATED QUESTIONS
Construct a ∆ABC such that:
CB = 6.5 cm, CA = 4.2 cm and BA = 51 cm
Construct a ∆ABC such that:
BC = 4 cm, AC = 5 cm and AB = 3.5 cm
Construct a ∆ ABC such that:
BC = 6 cm, AC = 5.7 cm and ∠ACB = 75°
Construct a ∆ ABC such that:
AB = 6.5 cm, AC = 5.8 cm and ∠A = 45°
Construct an isosceles Δ ABC such that:
Base BC = 4 cm and base angle = 30°. Measure the other two sides of the triangle.
Construct an isosceles ∆ ABC such that:
One of the equal sides = 6 cm and vertex angle = 45°. Measure the base angles.
Construct an equilateral Δ ABC such that:
AB = 5 cm. Draw the perpendicular bisectors of BC and AC. Let P be the point of intersection of these two bisectors. Measure PA, PB, and PC.
Construct a ∆ ABC such that AB = 6 cm, BC = 4.5 cm and AC = 5.5 cm. Construct a circumcircle of this triangle.
Construct an equilateral triangle ABC such that it's one side = 5.5 cm. Construct a circumcircle to this triangle.
Construct a ∆ PQR such that PQ = 6 cm, ∠QPR = 45° and angle PQR = 60°. Locate its incentre and then draw its incircle.
