Advertisements
Advertisements
Question
D(– 1, 8), E(4, – 2), F(– 5, – 3) are midpoints of sides BC, CA and AB of ΔABC Find: equations of sides of ΔABC.
Solution
Let A(x1, y1), B(x2, y2) and C(x3, y3) be the vertices of ΔABC.
Given, points D, E and F are midpoints of sides BC, CA and AB respectively of ΔABC.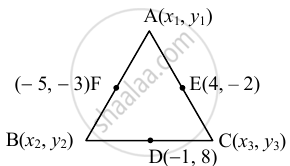
D = `((x_2 + x_3)/2, (y_2 + y_3)/2)`
∴ (–1 , 8) = `((x_2 + x_3)/2, (y_2 + y_3)/2)`
∴ x2 + x3 = – 2 ...(i)
and y2 + y3 = 16 ...(ii)
Also, E = `((x_1 + x_3)/2, (y_1 + y_3)/2)`
∴ (4, – 2) = `((x_1 + x_3)/2, (y_1 + y_3)/2)`
∴ x1 + x3 = 8 ...(iii)
and y1 + y3 = – 4 ...(iv)
Similarly, F = `((x_1 + x_2)/2, (y_1 + y_2)/2)`
∴ (– 5, – 3) = `((x_1 + x_2)/2, (y_1 + y_2)/2)`
∴ x1 + x2 = – 10 ...(v)
and y1 + y2 = – 6 ...(vi)
For x-coordinates:
Adding (i), (iii) and (v), we get
2x1 + 2x2 + 2x3 = – 4
∴ x1 + x2 + x3 = – 2 ...(vii)
Solving (i) and (vii), we get
x1 = 0
Solving (iii) and (vii), we get
x2 = – 10
Solving (v) and (vii), we get
x3 = 8
For y-coordinates:
Adding (ii), (iv) and (vi), we get
2y1 + 2y2 + 2y3 = 6
∴ y1 + y2 + y3 = 3 ...(viii)
Solving (ii) and (viii), we get
y1 = – 13
Solving (iv) and (viii), we get
y2 = 7
Solving (vi) and (viii), we get
y3 = 9
∴ Vertices of ΔABC are A.(0, – 13), B(– 10, 7), C(8, 9)
a. Equation of side AB is
`(y + 13)/(7 +13) = (x - 0)/(-10 - 0)`
∴ `(y + 13)/20 = x/(-10)`
∴ `(y + 13)/2` = – x
∴ 2x + y + 13 = 0
b. Equation of side BC is
`(y - 7)/(9 - 7) = (x + 10)/(8 + 10)`
∴ `(y - 7)/2 = (x + 10)/9`
∴ y – 7 = `(x + 10)/9`
∴ x – 9y + 73 = 0
c. Equation of side AC is
`(y + 13)/(9 + 13) = (x - 0)/(8 - 0)`
∴ `(y + 13)/22 = x/8`
∴ 8(y + 130 = 22x
∴ 4(y + 13) = 11x
∴ 11x – 4y – 52 = 0
APPEARS IN
RELATED QUESTIONS
Write the equation of the line: parallel to the X-axis and at a distance of 5 units from it and above it.
Write the equation of the line: parallel to the Y-axis and at a distance of 5 units from it and to the left of it.
Obtain the equation of the line: parallel to the X-axis and making an intercept of 3 units on the Y-axis.
Obtain the equation of the line: parallel to the Y-axis and making an intercept of 4 units on the X-axis.
Obtain the equation of the line containing the point: A(2, – 3) and parallel to the Y-axis.
Obtain the equation of the line containing the point: B(4, – 3) and parallel to the X-axis.
Show that the lines x – 2y – 7 = 0 and 2x − 4y + 5 = 0 are parallel to each other.
If the line 3x + 4y = p makes a triangle of area 24 square units with the co-ordinate axes, then find the value of p.
Find the co-ordinates of the circumcentre of the triangle whose vertices are A(– 2, 3), B(6, – 1), C(4, 3).
Find the distance between parallel lines 9x + 6y − 7 = 0 and 9x + 6y − 32 = 0.
Find the equation of the line passing through the point of intersection of lines x + y – 2 = 0 and 2x – 3y + 4 = 0 and making intercept 3 on the X-axis.
Which of the following lines passes through the origin?
Obtain the equation of the line which is: parallel to the X-axis and 3 units below it.
Obtain the equation of the line which is parallel to the Y-axis and 2 units to the left of it.
Obtain the equation of the line which is parallel to the X-axis and making an intercept of 5 on the Y-axis.
Obtain the equation of the line which is: parallel to the Y-axis and making an intercept of 3 on the X-axis.
Obtain the equation of the line containing the point: (2, 3) and parallel to the X−axis.
