Advertisements
Advertisements
Question
If the line 3x + 4y = p makes a triangle of area 24 square units with the co-ordinate axes, then find the value of p.
Solution
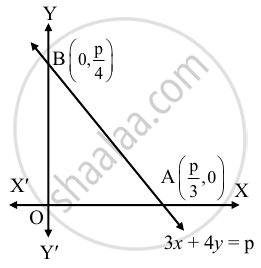
Let the line 3x + 4y = p cuts the X and Y-axes at points A and B respectively.
3x + 4y = p
∴ `(3x)/"p" + (4y)/"p"` = 1
∴ `x/("p"/3) + y/("p"/4)` = 1
This equation is of the form
`"x"/"a" + y/"b"` = 1.
with a = `"p"/3 and "b" = "p"/4`
∴ A ≡ `("a", 0) = ("p"/3 , 0) and "B" ≡ (0, "b") = (0, "p"/4)`
∴ OA = `"p"/3 and "OB" = "p"/4`
Given, A (Δ.OAB) = 24 sq. units
∴ `1/2 xx "OA" xx "OB"` = 24
∴ `1/2 xx "p"/3 xx "p"/4`= 24
∴ p2 = 576
∴ p = ± 24
APPEARS IN
RELATED QUESTIONS
Write the equation of the line: parallel to the X-axis and at a distance of 5 units from it and above it.
Write the equation of the line: parallel to the Y-axis and at a distance of 5 units from it and to the left of it.
Write the equation of the line: parallel to the X-axis and at a distance of 4 units from the point (−2, 3).
Obtain the equation of the line: parallel to the X-axis and making an intercept of 3 units on the Y-axis.
Obtain the equation of the line: parallel to the Y-axis and making an intercept of 4 units on the X-axis.
Obtain the equation of the line containing the point: A(2, – 3) and parallel to the Y-axis.
Obtain the equation of the line containing the point: B(4, – 3) and parallel to the X-axis.
Show that the lines x – 2y – 7 = 0 and 2x − 4y + 5 = 0 are parallel to each other.
Find the co-ordinates of the circumcentre of the triangle whose vertices are A(– 2, 3), B(6, – 1), C(4, 3).
Find the distance of the point A(– 2, 3) from the line 12x – 5y – 13 = 0.
Find the equation of the line passing through the point of intersection of lines x + y – 2 = 0 and 2x – 3y + 4 = 0 and making intercept 3 on the X-axis.
D(– 1, 8), E(4, – 2), F(– 5, – 3) are midpoints of sides BC, CA and AB of ΔABC Find: equations of sides of ΔABC.
Obtain the equation of the line which is: parallel to the X-axis and 3 units below it.
Obtain the equation of the line which is parallel to the Y-axis and 2 units to the left of it.
Obtain the equation of the line which is parallel to the X-axis and making an intercept of 5 on the Y-axis.
Obtain the equation of the line which is: parallel to the Y-axis and making an intercept of 3 on the X-axis.
Obtain the equation of the line containing the point: (2, 3) and parallel to the X−axis.
