Advertisements
Advertisements
Question
Derive an expression for electrostatic potential due to a point charge.
Solution
- Consider a positive charge ‘q’ kept fixed at the origin. Let P be a point at distance r from the charge ‘q’.

Electrostatic potential at a point P - The electric potential at the point P is
V = `int_∞^"r" (- vec"E")* "d"vec"r" = - int_∞^"r" vec"E" * vec"dr"` - Electric field due to positive point charge is
`vec"E" = 1/(4piε_0) "q"/"r"^2 hat"r"`
V = `- 1/(4piε_0) int_∞^"r" "q"/"r"^2 hat"r" * "d" vec"r"` - The infinitesimal displacement vector,
`"d"vec"r" = "dr" hat"r"` and using `hat "r" hat "r"` = 1, we have
V = `- 1/(4piε_0) int_∞^"r" "q"/"r"^2 hat"r" * "dr" hat"r" = - 1/(4piε_0) int_∞^"r" "q"/"r"^2 "dr"` - After the integration,
V = `- 1/(4piε_0) "q"{- 1/"r"}_∞^"r" = 1/(4piε_0) "q"/"r"` - Hence the electric potential due to a point charge q at a distance r is
V = `1/(4piε_0) "q"/"r"`
APPEARS IN
RELATED QUESTIONS
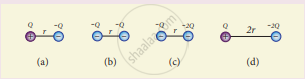
Rank the electrostatic potential energies for the given system of charges in increasing order.
An electric field `vec"E" = 10 xx hat"i"` exists in a certain region of space. Then the potential difference V = Vo – VA, where Vo is the potential at the origin and VA is the potential at x = 2 m is:
A thin conducting spherical shell of radius R has a charge Q which is uniformly distributed on its surface. The correct plot for electrostatic potential due to this spherical shell is
Two points A and B are maintained at a potential of 7 V and -4 V respectively. The work done in moving 50 electrons from A to B is ______.
What is an equipotential surface?
Give the relation between electric field and electric potential.
Obtain an expression for potential energy due to a collection of three point charges which are separated by finite distances.
Draw the free body diagram for the following charges as shown in the following figure.
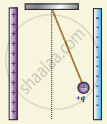
Draw the free body diagram for the following charges as shown in the following figure.

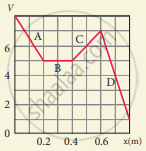
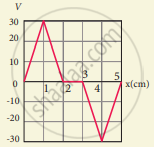
The electrostatic potential is given as a function of x in figure (a) and (b). Calculate the corresponding electric fields in regions A, B, C and D. Plot the electric field as a function of x for figure (b).