Advertisements
Advertisements
Question
Derive the formula for the volume of the frustum of a cone.
Solution
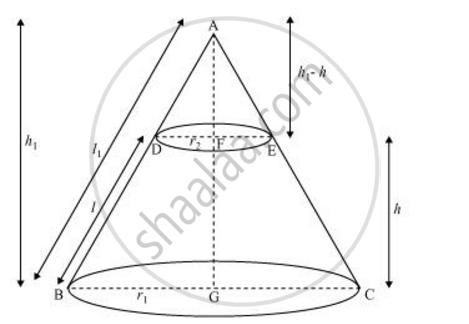
Let ABC be a cone. A frustum DECB is cut by a plane parallel to its base.
Let r1 and r2 be the radii of the ends of the frustum of the cone and h be the height of the frustum of the cone.
In ΔABG and ΔADF, DF||BG
∴ ΔABG ∼ ΔADF
DF/BG = AF/AG = AD/AB
`r_2/r_1 = (h_1-h)/h_1 = (l_1-l)/l_1`
`r_2/r_1 =1 -h/h_1 =1 -l/l_1`
`1-h/h_1= r_2/r_1`
`h/h_1 =1 -r_2/r_1 = (r_1-r_2)/r_1`
`h_1/h= r_1/(r_1-r_2)`
`h_1 = (r_1h)/(r_1-r_2)`
Volume of frustum of cone = Volume of cone ABC − Volume of cone ADE
`=1/3pir_1^2h_1 - 1/3pir_2^2(h_1-h)`
`=pi/3[r_1^2h_1-r_2^2(h_1-h)]`
`=pi/3[r_1^2((hr_1)/(r_1-r_2))-r_2^2((hr_1)/(r_1-r_2)-h)]`
`=pi/3[((hr_1^3)/(r_1-r_2))-r_2^2((hr_1-hr_1+hr_2)/(r_1-r_2))]`
`=pi/3[(hr_1^3)/(r_1-r_2)-(hr_2^3)/(r_1-r_2)]`
`=pi/3h[(r_1^3-r_2^3)/(r_1-r_2)]`
`=pi/3h[((r_1-r_2)(r_1^2+r_2^2+r_1r_2))/(r_1-r_2)]`
`= 1/3pih[r_1^2+r_2^2+r_1r_2]`
APPEARS IN
RELATED QUESTIONS
The height of a cone is 30 cm. From its topside a small cone is cut by a plane parallel to its base. If volume of smaller cone is `1/27` of the given cone, then at what height it is cut from its base?
Milk in a container, which is in the form of a frustum of a cone of height 30 cm and the radii of whose lower and upper circular ends are 20 cm and 40 cm respectively, is to be distributed in a camp for flood victims. If this milk is available at the rate of Rs 35 per litre and 880 litres of milk is needed daily for a camp, find how many such containers of milk are needed for a camp and what cost will it put on the donor agency for this. What value is indicated through this by the donor agency ?
An open metal bucket is in the shape of a frustum of a cone of height 21 cm with radii of its lower and upper ends as 10 cm and 20 cm respectively. Find the cost of milk which can completely fill the bucket at Rs. 30 per litre. `[User pi22/7]`
The surface area of a sphere is the same as the curved surface area of a cone having the radius of the base as 120 cm and height 160 cm. Find the radius of the sphere.
A frustum of a cone is 9 cm thick and the diameters of its circular ends are 28 cm and 4 cm. Find the volume and lateral surface area of the frustum.
(Take π = 22/7).
A bucket made up of a metal sheet is in the form of a frustum of a cone of height 16 cm and radii of its lower and upper ends are 8 cm and 20 cm, respectively. Find the cost of the bucket if the cost of metal sheet used is Rs 15 per 100 cm2.
A cone is cut by a plane parallel to its base and the upper part is removed. The part that is left is called

An open metal bucket is in the shape of a frustum of a cone, mounted on a hollow cylindrical base made of the same metallic sheet. The diameters of the two circular ends of the bucket are 45 cm and 25 cm, the total vertical height of the bucket is 40 cm and that of the cylindrical base is 6 cm. Find the area of the metallic sheet used to make the bucket. Also, find the volume of water the bucket can hold, in litres.
An oil funnel of the tin sheet consists of a cylindrical portion 10 cm long attached to a frustum of a cone. If the total height is 22 cm, the diameter of the cylindrical portion by 8 cm and the diameter of the top of the funnel be 18 cm, then find the area of the tin sheet required to make the funnel.
The shape of a glass (tumbler) (see figure) is usually in the form of ______.
