Advertisements
Advertisements
Question
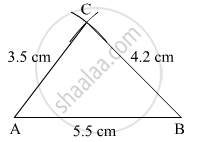
In ΔABC, l(AB) = 5.5 cm, l(BC) = 4.2 cm, l(AC) = 3.5 cm
Solution
Steps of construction:
- Draw a line AB = 5.5 cm.
- With A as a centre and 3.5 cm as the radius, draw an arc above the line AB.
- With B as the center and 4.2 cm as the radius, draw an arc cutting the previous drawn arc at point C.
- Join CA and CB.
Δ ABC is thus the required triangle.
RELATED QUESTIONS
Draw triangle with the measures given below.
In ∆FUN, l(FU) = 5 cm, l(UN) = 4.6 cm, m∠U = 110°
Construct a triangle of the measures given below.
In ∆SAT, l(AT) = 6.4 cm, m ∠A = 45°, m ∠T = 105°
Construct a triangle of the measures given below.
In ∆MNP, l(NP) = 5.2 cm, m∠N = 70°, m ∠P = 40°
Construct a triangle of the measures given below.
Construct ∆XYZ such that l(XY) = 3.7 cm, l(YZ) = 7.7 cm, l(XZ) = 6.3 cm.
Construct ∆PQR such that m∠P = 80°, m∠Q = 70°, l(QR) = 5.7 cm.
Construct a right-angled triangle in which: Side AB = 4.5 cm and hypotenuse AC = 7 cm
Construct an isosceles triangle in which: AB = AC, BC = 6 cm and ∠B = 75°
Construct an isosceles triangle in which: XY = XZ, YZ = 5.5 cm and ∠X = 60°
Construct a ΔABC, right-angled at B with a perimeter of 10 cm and one acute angle of 60°.
