Advertisements
Advertisements
Question
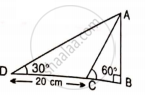
Find angle 'x' if :
Solution

The above figure we have
tan 60° = `(30)/"AD"`
`sqrt(3) = (30)/"AD"`
AD = `(30)/(sqrt(3)`
Again
sin x = `"AD"/(20)`
AD = 20 sin x
Now
20 sin x = `(30)/sqrt(3)`
sin x = `(30)/(20sqrt(3)`
sin x = `sqrt(3)/(2)`
sin x = sin 60°
x = 60°
APPEARS IN
RELATED QUESTIONS
Find AD, if :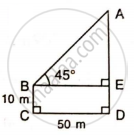
Find the lengths of diagonals AC and BD. Given AB = 60 cm and ∠ BAD = 60°.

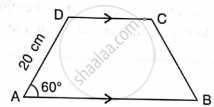
In trapezium ABCD, as shown, AB // DC, AD = DC = BC = 20 cm and ∠ A = 60°. Find: length of AB
In the given figure, AB and EC are parallel to each other. Sides AD and BC are 2 cm each and are perpendicular to AB.
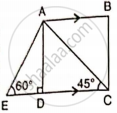
Given that ∠ AED = 60° and ∠ ACD = 45°. Calculate: AB.
In the given figure, AB and EC are parallel to each other. Sides AD and BC are 2 cm each and are perpendicular to AB.

Given that ∠AED = 60° and ∠ACD = 45°. Calculate: AC
Find: AD
In right-angled triangle ABC; ∠B = 90°. Find the magnitude of angle A, if:
BC is `sqrt(3)` times of AB.
A ladder is placed against a vertical tower. If the ladder makes an angle of 30° with the ground and reaches upto a height of 15 m of the tower; find length of the ladder.
A kite is attached to a 100 m long string. Find the greatest height reached by the kite when its string makes an angles of 60° with the level ground.
Find AB and BC, if: