Advertisements
Advertisements
Question
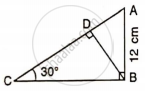
Find AB and BC, if:
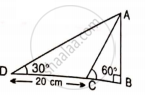
Solution
Let BC = x m
BD = BC + CD = (x + 20) cm
In ΔABD,
tan 30° = `"AB"/"BD"`
`(1)/(sqrt(3)) = "AB"/(x + 20)`
x + 20 = `sqrt(3)"AB"` ...(1)
In ΔABC
tan 60° = `"AB"/"BC"`
`sqrt(3) = "AB"/x`
x = `"AB"/sqrt(3)` ...(2)
From (1)
`"AB"/sqrt(3) + 20 = sqrt(3)"AB"`
AB + `20sqrt(3)` = 3AB
2AB = `20sqrt(3)`
2AB = `(20sqrt(3))/(2)`
AB = `10sqrt(3)`
AB = 17.32 cm
From (2)
x = `"AB"/sqrt(3)`
x = `(17.32)/sqrt(3)`
x = 10 cm
Therefore BC = x = 10 cm
Therefore, AB = 17.32 cm, BC = 10 cm.
APPEARS IN
RELATED QUESTIONS
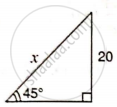
Find 'x', if:
Find angle 'x' if :
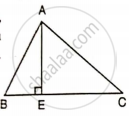
In the given figure, ∠B = 60°, AB = 16 cm and BC = 23 cm,
Calculate:
- BE
- AC
Find: BC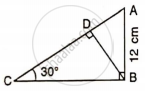
Find: AD
In right-angled triangle ABC; ∠ B = 90°. Find the magnitude of angle A, if: AB is √3 times of BC.
A kite is attached to a 100 m long string. Find the greatest height reached by the kite when its string makes an angles of 60° with the level ground.
Find AB and BC, if:
If tan x° = `(5)/(12)`,
tan y° = `(3)/(4)` and AB = 48 m; find the length of CD.
The perimeter of a rhombus is 96 cm and obtuse angle of it is 120°. Find the lengths of its diagonals.
