Question

Find the lengths of the medians of a  ΔABC whose vertices are A(0,-1) , B(2,1) and C (0.3).

Answer 1

The vertices of ABC  A(0,-1) , B(2,1) and C (0.3)

Let AD, BE and CF be the medians of Δ ABC.

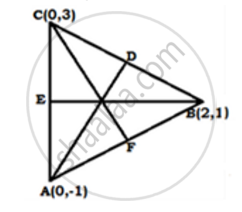
Let D be the midpoint of BC. So, the coordinates of D ar

`D ((2+0)/2 , (1+3)/2)  i.e  D (2/2 , 4/2)  i.e D (1,2)`

Let E be the midpoint of AC. So the coordinate of E are

 `E ((0+0)/2 , (-1+3)/2)  i.e . E (0/2,0/2)  i.e  E  (0,1) `

Let F be the midpoint of AB. So, the coordinates of F are

 ` F ((0+2)/2  , (-1+1)/2)  i.e  F (2/2 , 0/2) i.e  F  (1,0) `

 `AD = sqrt((1-0)^2 +(2-(-1))^2) = sqrt((1)^2 +(3)^2) = sqrt(1+9) = sqrt(10)  units`

` BE = sqrt((0-2)^2 +(1-1)^2) = sqrt((-2)^2 +(0)^2) = sqrt(4+0) = sqrt(4)= 2  units`

`CF = sqrt((1-0)^2 +(0-3)^2) = sqrt((1)^2 +(-3)^2) = sqrt(1+9) = sqrt(10)  units`

`"Therefore, the lengths of the medians:" AD = sqrt(10) units . BE=2 units and CF = sqrt(10)  units .`