Advertisements
Advertisements
Question
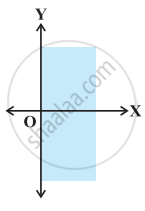
Find the linear inequations for which the solution set is the shaded region given in Fig. 15.42
Solution
Considering the line x + y = 4, we find that the shaded region and the origin (0, 0) are on the same side of this line and (0, 0) does not satisfy the inequation x + y \[\leq\] 4Considering the line y = 3, we find that the shaded region and the origin (0, 0) are on the same side of this line and (0, 0) satisfies the inequation y\[\leq\]3 So, the corresponding inequation is y\[\leq\]3Considering the line x = 3, we find that the shaded region and the origin (0, 0) are on the same side of this line and (0, 0) satisfies the inequation x\[\leq\] 3 So, the corresponding inequation is x 3 Considering the line x + 5y = 4, we find that the shaded region and the origin (0, 0) are on the opposite side of this line and (0, 0) does not satisfy the inequation x + 5y\[\geq 4\]So, the corresponding inequation is x + 5y \[\geq 4\] Considering the line 6x + 2y = 8, we find that the shaded region and the origin (0, 0) are on the opposite side of this line and (0, 0) does not satisfy the inequation 6x + 2y\[\geq 8\]So, the corresponding inequation is 6x + 2y\[\geq 8\]Also the shaded region is in the first quadrant. Therefore, we must have \[x \geq 0 \text{ and } y \geq 0\]
Thus, the linear inequations comprising the given solution set are given below:
x + y\[\leq\]4, y\[\leq\]3, x\[\leq\]3, x + 5y\[\geq 4\]6x + 2y\[\geq 8\]\[x \geq 0 \text{ and } y \geq 0\]
APPEARS IN
RELATED QUESTIONS
Solve the given inequality graphically in two-dimensional plane: x + y < 5
Solve the given inequality graphically in two-dimensional plane: 2x + y ≥ 6
Solve the given inequality graphically in two-dimensional plane: 3x + 4y ≤ 12
Solve the given inequality graphically in two-dimensional plane: y + 8 ≥ 2x
Solve the given inequality graphically in two-dimensional plane: x – y ≤ 2
Solve the given inequality graphically in two-dimensional plane: 3y – 5x < 30
Solve the given inequality graphically in two-dimensional plane: y < –2
Solve the inequalities and represent the solution graphically on number line:
5x + 1 > –24, 5x – 1 < 24
Solve the inequality and represent the solution graphically on number line:
2(x – 1) < x + 5, 3(x + 2) > 2 – x
Solve the inequalities and represent the solution graphically on number line:
5(2x – 7) – 3(2x + 3) ≤ 0, 2x + 19 ≤ 6x + 47
A solution is to be kept between 68° F and 77° F. What is the range in temperature in degree Celsius (C) if the Celsius/Fahrenheit (F) conversion formula is given by `F= 9/8` C + 32?
A solution of 8% boric acid is to be diluted by adding a 2% boric acid solution to it. The resulting mixture is to be more than 4% but less than 6% boric acid. If we have 640 litres of the 8% solution, how many litres of the 2% solution will have to be added?
How many litres of water will have to be added to 1125 litres of the 45% solution of acid so that the resulting mixture will contain more than 25% but less than 30% acid content?
Solve the following systems of linear inequation graphically:
2x + 3y ≤ 6, 3x + 2y ≤ 6, x ≥ 0, y ≥ 0
Solve the following systems of linear inequation graphically:
2x + 3y ≤ 6, x + 4y ≤ 4, x ≥ 0, y ≥ 0
Solve the following systems of linear inequations graphically:
2x + 3y ≤ 35, y ≥ 3, x ≥ 2, x ≥ 0, y ≥ 0
Show that the solution set of the following linear inequations is empty set:
x + 2y ≤ 3, 3x + 4y ≥ 12, y ≥ 1, x ≥ 0, y ≥ 0
Find the linear inequations for which the shaded area in Fig. 15.41 is the solution set. Draw the diagram of the solution set of the linear inequations:
Solve the following systems of inequations graphically:
2x + y ≥ 8, x + 2y ≥ 8, x + y ≤ 6
Solve the following systems of inequations graphically:
x + 2y ≤ 40, 3x + y ≥ 30, 4x + 3y ≥ 60, x ≥ 0, y ≥ 0
Solve the following systems of inequations graphically:
5x + y ≥ 10, 2x + 2y ≥ 12, x + 4y ≥ 12, x ≥ 0, y ≥ 0
Show that the following system of linear equations has no solution:
\[x + 2y \leq 3, 3x + 4y \geq 12, x \geq 0, y \geq 1\]
Mark the correct alternative in each of the following:
If x\[<\]7, then
Write the solution of the inequation\[\frac{x^2}{x - 2} > 0\]
Find the linear inequalities for which the shaded region in the given figure is the solution set.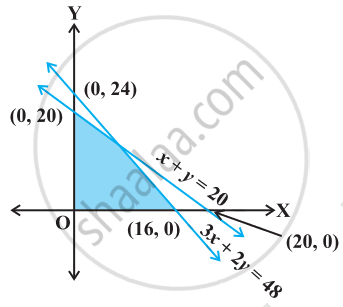
State which of the following statement is True or False.
If x < y and b < 0, then `x/"b" < y/"b"`
State which of the following statement is True or False.
If xy > 0, then x < 0 and y < 0
Graph of x < 3 is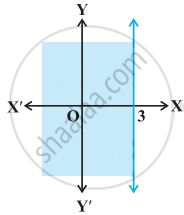
Graph of x ≥ 0 is