Question

Find the mutual inductance between the circular coil and the loop shown in figure.

Answer 1

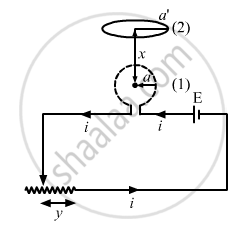
The magnetic field due to coil 1 at the centre of coil 2 is given by

\[B = \frac{\mu_0 Ni a^2}{2 ( a^2 + x^2 )^{3/2}}\]

The flux linked with coil 2 is given by

\[\phi = B . A' = \frac{\mu_0 Ni a^2}{2  ( a^2 + x^2 )^{3/2}}\pi a '^2\]

Now, let y be the distance of the sliding contact from its left end.

Given:-

\[v = \frac{dy}{dt}\]

Total resistance of the rheostat = R

When the distance of the sliding contact from the left end is y, the resistance of the rheostat is given by

\[r' = \frac{R}{L}y\]

The current in the coil is the function of distance y travelled by the sliding contact of the rheostat. It is given by

\[i = \frac{E}{\left( \frac{R}{L}y + r \right)}\]

The magnitude of the emf induced can be calculated as:-

\[e = \frac{d\phi}{dt} = \frac{\mu_0 N a^2 a '^2 \pi}{2  ( a^2 + x^2 )^{3/2}}\frac{di}{dt}\]

\[e = \frac{\mu_0 N \pi a^2 a '^2}{2 ( a^2 + x^2 )^{3/2}}\frac{d}{dt}\frac{E}{\left( \frac{R}{L}y + r \right)}\]

\[e = \frac{\mu_0 N \pi a^2 a '^2}{2 ( a^2 + x^2 )^{3/2}}\left[ E\frac{\left( - \frac{R}{L}v \right)}{\left( \frac{R}{L}y + r \right)^2} \right]\]

emf induced,

\[e = \frac{\mu_0 N \pi a^2 a '^2}{2 ( a^2 + x^2 )^{3/2}}\left[ E\frac{\left( - \frac{R}{L}v \right)}{\left( \frac{R}{L}y + r \right)^2} \right]\]

The emf induced in the coil can also be given as:-

\[\frac{di}{dt} = \left[ E\frac{\left( - \frac{R}{L}v \right)}{\left( \frac{R}{L}y + r \right)^2} \right]\]

\[e = M\frac{di}{dt}  ,   \frac{di}{dt} = \left[ E\frac{\left( - \frac{R}{L}v \right)}{\left( \frac{R}{L}y + r \right)^2} \right]\]

\[M = \frac{e}{\frac{di}{dt}} = \frac{N \mu_0 \pi a^2 a '^2}{2( a^2 + x^2 )^{3/2}}\]