Advertisements
Advertisements
Question
Find the radius of the circular base of the cone , if its volume is 154 cm3 and the perpendicular height is 12 cm
Solution
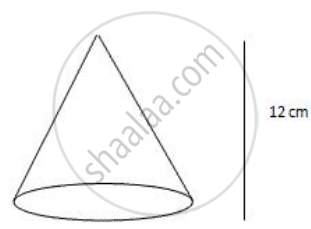
Volume of the cone = 154 cm3
⇒ `1/3 xx (pir^2) xx h = 154`
⇒ `1/3 xx (pir^2) xx 12 = 154`
⇒ `r^2 = (154 xx 3 xx 7)/(12 xx 22)`
⇒ `r^2 = 12.25`
⇒ r = 3.5 cm
Radius of the circular base of the cone is 3.5 cm
APPEARS IN
RELATED QUESTIONS
Find the curved surface area of a cone, if its slant height is 60 cm and the radius of its base is 21 cm.
The radius of a cone is 7 cm and area of curved surface is 176 `cm^2`. Find the slant height.
The area of the curved surface of a cone is 60 cm2. If the slant height of the cone be 8 cm, find the radius of the base?
If the radius of the base of a cone is halved, keeping the height same, what is the ratio of the volume of the reduced cone to that of the original cone?
A heap of wheat is in the form of a cone of diameter 9 m and height 3.5 m. Find its volume. How much canvas cloth is required to just cover the heap? (Use 𝜋 = 3.14).
The circumference of the base of a 12 m high conical tent is 66 m. Find the volume of the air contained in it.
Find what length of canvas, 1.5 m in width, is required to make a conical tent 48 m in diameter and 7 m in height. Given that 10% of the canvas is used in folds and stitchings. Also, find the cost of the canvas at the rate of Rs. 24 per metre.
The radii of the bases of two solid right circular cones of same height are r1 and r2 respectively. The cones are melted and recast into a solid sphere of radius R. Find the height of each cone in terms r1, r2 and R.
A solid metallic hemisphere of diameter 28 cm is melted and recast into a number of identical solid cones, each of diameter 14 cm and height 8 cm. Find the number of cones so formed.
A cone and a hemisphere have the same base and the same height. Find the ratio between their volumes.
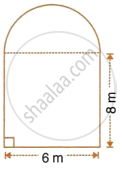
The cross-section of a railway tunnel is a rectangle 6 m broad and 8 m high surmounted by a semi-circle as shown in the figure. The tunnel is 35 m long. Find the cost of plastering the internal surface of the tunnel (excluding the floor) at the rate of Rs. 2.25 per m2.
Curved surface area of a cone is 251.2 cm2 and radius of its base is 8 cm. Find its slant height and perpendicular height. (π = 3.14)
Surface area of a cone is 188.4 sq.cm and its slant height is 10 cm. Find its perpendicular height ( π= 3.14)
Find the curved surface area of a cone whose height is 8 cm and base diameter is 12 cm .
Find the height of the cone whose base radius is 5 cm and volume is 75π cm3.
A buoy is made in the form of a hemisphere surmounted by a right circular cone whose circular base coincides with the plane surface of the hemisphere. The radius of the base of the cone is 3.5 m and its volume is two-third the volume of hemisphere. Calculate the height of the cone and the surface area of the buoy, correct to two decimal places.
The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm and the other dimensions are as shown. Calculate: the total volume of the solid.
Water flows at the rate of 10 m per minute through a cylindrical pipe 5 mm of diameter. How much time would it take to fill a conical vessel whose diameter at he surface is 40 cm and depth is 24 cm?
How many square metres of canvas is required for a conical tent whose height is 3.5 m and the radius of the base is 12 m?
