Advertisements
Advertisements
Question
Find the coordinates of the circumcentre of a triangle whose vertices are (–3, 1), (0, –2) and (1, 3).
Solution
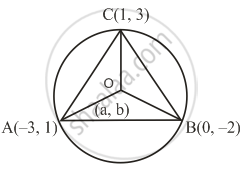
Let A(−3, 1), B(0, −2), C(1, 3) and the circumference O(a, b).
The distance of point O will be equal from A, B and C.
OA = OC ...(Radii of the same circle)
∴ `sqrt([a - (-3)]^2 + (b - 1)^2) = sqrt((a - 1)^2 + (b - 3)^2)` ...(Distance formula)
Squaring both the sides,
`(a + 3)^2 + (b - 1)^2 = (a - 1)^2 + (b - 3)^2`
`∴ cancela^2 + 6a +cancel9 + cancelb^2 − 2b + cancel1 = cancela^2 − 2a + cancel1 + cancelb^2 − 6b + cancel9`
∴ 6a − 2b = −2a − 6b
∴ 6a + 2a = − 6b + 2b
∴ 8a = −4b
∴ 8a + 4b = 0
∴ 4(2a + b) = 0
∴ 2a + b = 0 ....(I)
OB = OC ...(Radii of the same circle)
∴ `sqrt((a - 0)^2 + [b - (- 2)]^2) = sqrt((a - 1)^2 + (b - 3)^2)` ...(Distance formula)
Squaring both the sides,
`(a - 0)^2 + (b + 2)^2 = (a - 1)^2 + (b - 3)^2`
`∴ cancela^2 + cancelb^2 + 4b + 4 = cancela^2 − 2a + 1 + cancelb^2 − 6b + 9`
∴ 4b + 4 = − 2a − 6b + 10
∴ 4b + 2a + 6b = 10 - 4
∴ 2a + 10b = 6 ...(II)
Subtracting I from II, we get,
\[\begin{array}{1}
\phantom{\texttt{}}\texttt{2a + b = 0}\\ \phantom{\texttt{}}\texttt{- 2a + 10b = 6}\\ \hline\phantom{\texttt{}}\texttt{(-) (-) (-)}\\ \hline \end{array}\]
∴ - 9b = - 6
∴ b = `(-6)/(-9)`
∴ b = `2/3`
Substituting b = `2/3` in equation I, we get,
∴ 2a + b = 0
∴ 2a + `2/3` = 0
∴ 2a = `-2/3`
∴ a = `-2/3 xx 1/2`
∴ a = `(-1)/3`
Coordinates of the circumcentre are `((-1)/3, 2/3)`.
RELATED QUESTIONS
Prove that the points (3, 0), (6, 4) and (-1, 3) are the vertices of a right-angled isosceles triangle.
Find a point on y-axis which is equidistant from the points (5, -2) and (-3, 2).
Prove that the points A(-4,-1), B(-2, 4), C(4, 0) and D(2, 3) are the vertices of a rectangle.
If the points p (x , y) is point equidistant from the points A (5,1)and B ( -1,5) , Prove that 3x=2y
Find the co-ordinates of the point which divides the join of A(-5, 11) and B(4,-7) in the ratio 7 : 2
In what ratio is the line segment joining A(2, -3) and B(5, 6) divide by the x-axis? Also, find the coordinates of the pint of division.
Find the coordinates of the centre of the circle passing through the points P(6, –6), Q(3, –7) and R (3, 3).
Find the area of the quadrilateral ABCD, whose vertices are A(−3, −1), B (−2, −4), C(4, − 1) and D (3, 4).
The co-ordinates of point A and B are 4 and -8 respectively. Find d(A, B).
If P ( 9a -2 , - b) divides the line segment joining A (3a + 1 , - 3 ) and B (8a, 5) in the ratio 3 : 1 , find the values of a and b .
If (a,b) is the mid-point of the line segment joining the points A (10, - 6) , B (k,4) and a - 2b = 18 , find the value of k and the distance AB.
Points P, Q, R and S divides the line segment joining A(1, 2) and B(6, 7) in 5 equal parts. Find the coordinates of the points P, Q and R.
If three points (x1, y1) (x2, y2), (x3, y3) lie on the same line, prove that \[\frac{y_2 - y_3}{x_2 x_3} + \frac{y_3 - y_1}{x_3 x_1} + \frac{y_1 - y_2}{x_1 x_2} = 0\]
The distance between the points (a cos 25°, 0) and (0, a cos 65°) is
The ratio in which the x-axis divides the segment joining (3, 6) and (12, −3) is
If the points(x, 4) lies on a circle whose centre is at the origin and radius is 5, then x =
The line segment joining the points (3, -1) and (-6, 5) is trisected. The coordinates of point of trisection are ______.
Points (1, – 1), (2, – 2), (4, – 5), (– 3, – 4) ______.
Find the coordinates of the point whose ordinate is – 4 and which lies on y-axis.
Statement A (Assertion): If the coordinates of the mid-points of the sides AB and AC of ∆ABC are D(3, 5) and E(–3, –3) respectively, then BC = 20 units.
Statement R (Reason): The line joining the mid-points of two sides of a triangle is parallel to the third side and equal to half of it.
