Advertisements
Advertisements
Question
Find the equation of a line parallel to 2y = 6x + 7 and passing through (–1, 1).
Solution
Given the equation of a line,
2y = 6x + 7
Or y = `3x + 7/2`
Here, m = 3
The equation of a line with slope, m = 3 and passing through (–1, 1) is:
(y – y1) = m(x – x1)
`\implies` (y – 1) = m(x + 1)
`\implies` y – 1 = 3x + 3
`\implies` y = 3x + 3 + 1
`\implies` y = 3x + 4, is the required equation
APPEARS IN
RELATED QUESTIONS
The point (−3, 2) lies on the line ax + 3y + 6 = 0, calculate the value of a.
Find the equation of a line whose : y-intercept = 2 and slope = 3
Find the equation of the line with x-intercept 5 and a point on it (–3, 2).
The line through P (5, 3) intersects y-axis at Q.
write the equation of the line
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the co-ordinates of the centroid of triangle ABC.
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the equation of a line, through the centroid and parallel to AB.
Find the equation of a line passing through (3, – 2) and perpendicular to the line.
x - 3y + 5 = 0.
Find the equation of the straight line perpendicular to 5x – 2y = 8 and which passes through the mid-point of the line segment joining (2, 3) and (4, 5).
Find the equation of a straight line which cuts an intercept – 2 units from Y-axis and being equally inclined to the axis.
In the adjoining figure, write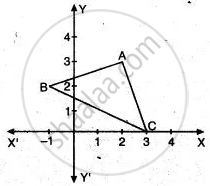
(i) The coordinates of A, B and C.
(ii) The equation of the line through A and | | to BC.
