Advertisements
Advertisements
Question
Find the equation of line through the intersection of lines 2x – y = 1 and 3x + 2y = –9 and making an angle of 30° with positive direction of x-axis.
Solution
Since the line passing through the x-axis makes an angle of 30° with the positive direction of the x-axis.
The slope of the line is given by tan `30^circ = 1/sqrt(3)`
The intersection of the lines 2x – y = 1 and 3x + 2y = –9 is given by solving the equation simultaneously.
So, multiplying equation 2x – y = 1 by 2, we get
4x – 2y = 2
Now add this resulting to the second equation 3x + 2y = –9
`=>` 7x = –7
`=>` x = –1
Substituting the value of x ∈ 2x – y = 1, we get
y = –3
Thus, the intersection of the lines is (–1, –3).
To find the equation of the required line,
We use the slope-point form, so we get
`y - (-3) = 1/sqrt(3)[x - (1)]`
i.e. `y + 3 = 1/sqrt(3)(x + 1)`
i.e `y = x/sqrt(3) + 1/sqrt(3) - 3`
APPEARS IN
RELATED QUESTIONS
A (5, 4), B (-3, -2) and C (1, -8) are the vertices of a triangle ABC. Find the equations of the median AD and line parallel to AC passing through the point B.
Write the equation of each of the following lines:
- The x-axis and the y-axis.
- The line passing through the origin and the point (-3, 5).
- The line passing through the point (-3, 4) and parallel to X-axis.
Find the slope and y-intercept of the line:
3x – 4y = 5
Find the equation of the line passing through (−2, 1) and perpendicular to 4x + 5y = 6.
- Write down the equation of the line AB, through (3, 2) and perpendicular to the line 2y = 3x + 5.
- AB meets the x-axis at A and the y-axis at B. Write down the co-ordinates of A and B. Calculate the area of triangle OAB, where O is the origin.
The points A, B and C are (4, 0), (2, 2) and (0, 6) respectively. Find the equations of AB and BC. If AB cuts the y-axis at P and BC cuts the x-axis at Q, find the co-ordinates of P and Q.
Show that points P(2, –2), Q(7, 3), R(11, –1) and S (6, –6) are vertices of a parallelogram.
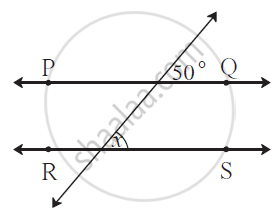
In the figure, line PQ || line RS. Using the information given
in the figure find the value of x.
In the figure, given, ABC is a triangle and BC is parallel to the y-axis. AB and AC intersect the y-axis at P and Q respectively.

- Write the co-ordinates of A.
- Find the length of AB and AC.
- Find the radio in which Q divides AC.
- Find the equation of the line AC.
A line segment joining P(2, –3) and Q(0, –1) is cut by the x-axis at the point R. A line AB cuts the y-axis at T(0, 6) and is perpendicular to PQ at S.
Find the:
- equation of line PQ
- equation of line AB
- coordinates of points R and S.
