Advertisements
Advertisements
Question
Show that points P(2, –2), Q(7, 3), R(11, –1) and S (6, –6) are vertices of a parallelogram.
Solution
The given points are P(2, –2), Q(7, 3), R(11, –1) and S(6, –6).
`"Distance between" = sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)`
By distance formula,
PQ = `sqrt((7 - 2)^2 + [3 - ( - 2)]^2)`
∴ PQ = `sqrt((7 - 2)^2 + (3 + 2)^2)`
∴ PQ = `sqrt((5)^2 + (5)^2)`
∴ PQ = `sqrt(25 + 25)`
∴ PQ = `sqrt(50)`
∴ PQ = `sqrt(25 × 2)`
∴ PQ = `5sqrt(2)` ...(1)
QR = `sqrt((11 - 7)^2 + (-1 - 3)^2)`
∴ QR = `sqrt((4)^2 + (-4)^2)`
∴ QR = `sqrt(16 + 16)`
∴ QR = `sqrt(32)`
∴ QR = `sqrt(16 × 2)`
∴ QR = `4sqrt(2)` ...(2)
RS = `sqrt((6 - 11)^2 + [-6 - (- 1)]^2)`
∴ RS = `sqrt((- 5)^2 + (-6 + 1)^2)`
∴ RS = `sqrt((- 5)^2 + (-5)^2)`
∴ RS = `sqrt(25 + 25)`
∴ RS = `sqrt(50)`
∴ RS = `sqrt(25 × 2)`
∴ RS = `5sqrt(2)` ...(3)
PS = `sqrt((6 - 2)^2 + [-6 - (- 2)]^2)`
∴ PS = `sqrt((6 - 2)^2 + [-6 + 2]^2)`
∴ PS = `sqrt((4)^2 + (-4)^2)`
∴ PS = `sqrt(16 + 16)`
∴ PS = `sqrt(32)`
∴ PS = `sqrt(16 × 2)`
∴ PS = `4sqrt(2)` ...(4)
In □ PQRS,
PQ = RS ...[From (1) and (3)]
QR = PS ...[From (2) and (4)]
A quadrilateral is a parallelogram, if both the pairs of its opposite sides are congruent.
Checking for slopes,
Slope of a line between two points (x1, y1) and (x2, y2) is
m = `(y_2 - y_1)/(x_2 - x_1)`
Slope PQ = `(7 - 2)/[3 - (- 2)] = 1`
Slope QR = `(11 - 7)/[- 1 - 3] = - 1`
Slope RS = `(6 - 11)/[- 6 - (- 1)] = 1`
Slope SP = `(6 - 2)/[- 6 - (- 2)] = - 1`
As PQ = RS and their slope = 1 And QR = SP and their slope = -1.
∴ □ PQRS is parallelogram.
∴ P, Q, R, and S are vertices of a parallelogram.
APPEARS IN
RELATED QUESTIONS
Write the equation of the line passing through the pair of points (2, 3) and (4, 7) in the form of y = mx + c.
A (5, 4), B (-3, -2) and C (1, -8) are the vertices of a triangle ABC. Find the equations of the median AD and line parallel to AC passing through the point B.
If (4,-3) is a point on the line AB and slope of the line is (-2), write the equation of the line AB.
Find the slope and y-intercept of the line:
3x – 4y = 5
The equation of a line is x – y = 4. Find its slope and y-intercept. Also, find its inclination.
Is the line 3x + 4y + 7 = 0 perpendicular to the line 28x – 21y + 50 = 0?
Is the line x – 3y = 4 perpendicular to the line 3x – y = 7?
Is the line 3x + 2y = 5 parallel to the line x + 2y = 1?
Find the equation of the line passing through (−2, 1) and perpendicular to 4x + 5y = 6.
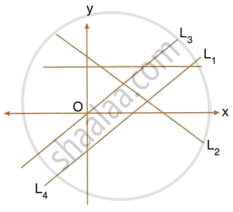
Match the equations A, B, C and D with the lines L1, L2, L3 and L4, whose graphs are roughly drawn in the given diagram.
A ≡ y = 2x;
B ≡ y – 2x + 2 = 0;
C ≡ 3x + 2y = 6;
D ≡ y = 2
Find the equation of the line which is perpendicular to the line `x/a - y/b = 1` at the point where this line meets y-axis.
A (5, 4), B (–3,–2) and C (1,–8) are the vertices of a triangle ABC. Find the equation of median AD and line parallel to AB passing through point C.
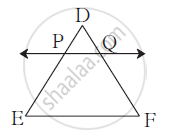
In Δ DEF, line PQ || side EF, If DP = 2.4,
PE = 7.2, DQ = 1 then find QF.
Prove that :
“If a line parallel to a side of a triangle intersects the remaining sides in two distince points, then the line divides the sides in the same proportion.”
Find:

- equation of AB
- equation of CD
If (4,-3) is a point on line 5x +8y = c, find the value of c.
Find the equation of the line passing through the points (4,-5) and (-1,-2).
