Advertisements
Advertisements
Question
Find:

- equation of AB
- equation of CD
Solution
i. Slope of AB = `(3 - 4)/(3 - (-5)) = -1/8`
∴ Equation of AB is given by
`y - 4 = -1/8(x - (-5))`
8y – 32 = –(x + 5)
8y – 32 = –x – 5
x + 8y = 27
ii. AB and CD are perpendicular to each other.
Thus, product of their slope = –1
Slope of AB × Slope of CD = –1
`=>` Slope of CD = 8
Now, from graph we have coordinates of D = (–3, 0)
∴ Equation of line CD is given by
y – y1 = m(x – x1)
y – 0 = 8(x – 3)
y = 8(x + 3)
y = 8x + 24
APPEARS IN
RELATED QUESTIONS
Write the equation of each of the following lines:
- The x-axis and the y-axis.
- The line passing through the origin and the point (-3, 5).
- The line passing through the point (-3, 4) and parallel to X-axis.
In ΔABC, A(3, 5), B(7, 8) and C(1, –10). Find the equation of the median through A.
Given 3x + 2y + 4 = 0
(i) express the equation in the form y = mx + c
(ii) Find the slope and y-intercept of the line 3x + 2y + 4 = 0
Find the slope and y-intercept of the line:
3x – 4y = 5
Is the line x – 3y = 4 perpendicular to the line 3x – y = 7?
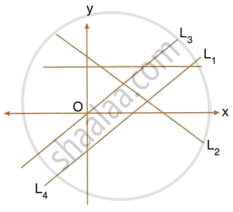
Match the equations A, B, C and D with the lines L1, L2, L3 and L4, whose graphs are roughly drawn in the given diagram.
A ≡ y = 2x;
B ≡ y – 2x + 2 = 0;
C ≡ 3x + 2y = 6;
D ≡ y = 2
Find the point on the X–axis which is equidistant from A(–3, 4) and B(1, –4).
Prove that :
“If a line parallel to a side of a triangle intersects the remaining sides in two distince points, then the line divides the sides in the same proportion.”
A straight line passes through the points P(–1, 4) and Q(5, –2). It intersects x-axis at point A and y-axis at point B. M is the mid-point of the line segment AB. Find:
- the equation of the line.
- the co-ordinates of point A and B.
- the co-ordinates of point M.
A line segment joining P(2, –3) and Q(0, –1) is cut by the x-axis at the point R. A line AB cuts the y-axis at T(0, 6) and is perpendicular to PQ at S.
Find the:
- equation of line PQ
- equation of line AB
- coordinates of points R and S.
