Advertisements
Advertisements
Question
Prove that :
“If a line parallel to a side of a triangle intersects the remaining sides in two distince points, then the line divides the sides in the same proportion.”
Solution
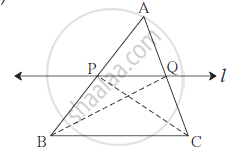
Given :In ΔABC line l || Side BC line l intersects side AB and side AC in P and Q respectively.
To prove : `(AP)/(PB) = (AQ)/(QC)`
Construction : Draw seg PC and seg QB.
Proof : `(A(APQ))/(A(PQB)) = (AP)/(PB)` ...... (I) (Areas are in proportion to the bases) `(A(APQ))/(A(PQB)) = (AQ)/(QC)` ....... (II) (Areas are in proportion to the bases) Δ PQB and Δ PQC have the same base PQ and PQ || BC,
their height is also same.
∴ A(Δ PQB) = A(Δ PQC) ..... (III)
∴` (A(APQ))/(A(PQB)) =( A(APQ))/(A(PQC))` ........ from ((I), (II) and (III)
∴ `(AP)/(PB) = (AQ)/(QC)` ........ from (I) , (II)
APPEARS IN
RELATED QUESTIONS
Write the equation of the line passing through the pair of points (2, 3) and (4, 7) in the form of y = mx + c.
Write the equation of each of the following lines:
- The x-axis and the y-axis.
- The line passing through the origin and the point (-3, 5).
- The line passing through the point (-3, 4) and parallel to X-axis.
Given 3x + 2y + 4 = 0
(i) express the equation in the form y = mx + c
(ii) Find the slope and y-intercept of the line 3x + 2y + 4 = 0
Find the slope and y-intercept of the line:
ax – by = 0
Find the slope and y-intercept of the line:
3x – 4y = 5
The equation of a line is x – y = 4. Find its slope and y-intercept. Also, find its inclination.
Is the line x – 3y = 4 perpendicular to the line 3x – y = 7?
Find the equation of the perpendicular bisector of the line segment obtained on joining the points (6, −3) and (0, 3).
A = (7, −2) and C = (−1, −6) are the vertices of square ABCD. Find the equations of diagonals AC and BD.
Find the equation of the line which is perpendicular to the line `x/a - y/b = 1` at the point where this line meets y-axis.
Find the point on the X–axis which is equidistant from A(–3, 4) and B(1, –4).
Verify that points P(–2, 2), Q(2, 2) and R(2, 7) are vertices of a right angled triangle.
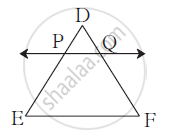
In Δ DEF, line PQ || side EF, If DP = 2.4,
PE = 7.2, DQ = 1 then find QF.
Find the equation of the line that has x-intercept = –3 and is perpendicular to 3x + 5y = 1.
Find the equation of the line through the points A(–1, 3) and B(0, 2). Hence, show that the point A, B and C(1, 1) are collinear.
Three vertices of a parallelogram ABCD taken in order are A(3, 6), B(5, 10) and C(3, 2), find:
- the co-ordinates of the fourth vertex D.
- length of diagonal BD.
- equation of side AB of the parallelogram ABCD.
Find the equation of the line passing through the points (4,-5) and (-1,-2).
