Advertisements
Advertisements
Question
A = (7, −2) and C = (−1, −6) are the vertices of square ABCD. Find the equations of diagonals AC and BD.
Solution
We know that in a square, diagonals bisect each other at right angle.
Let O be the point of intersection of the diagonals AC and BD.
Co-ordinates of O are
`((7 - 1)/2, (-2 - 6)/2) = (3, -4)`
Slope of AC = `(-6 + 2)/(-1 - 7) = (-4)/-8 = 1/2`
For line AC:
Slope = m = `1/2`, (x1, y1) = (7, –2)
Equation of the line AC is
y – y1 = m(x – x1)
`y + 2 =1/2 (x - 7)`
2y + 4 = x – 7
2y = x – 11
For line BD:
Slope = m = `(-1)/("slope of AC") = (-1)/(1/2)= -2`,
(x1, y1) = (3, −4)
Equation of the line BD is
y − y1 = m(x − x1)
y + 4 = −2(x − 3)
y + 4 = −2x + 6
2x + y = 2
APPEARS IN
RELATED QUESTIONS
If (4,-3) is a point on the line AB and slope of the line is (-2), write the equation of the line AB.
In ΔABC, A(3, 5), B(7, 8) and C(1, –10). Find the equation of the median through A.
The line 4x − 3y + 12 = 0 meets x-axis at A. Write the co-ordinates of A. Determine the equation of the line through A and perpendicular to 4x – 3y + 12 = 0.
The point P is the foot of perpendicular from A(−5, 7) to the line whose equation is 2x – 3y + 18 = 0. Determine :
- the equation of the line AP.
- the co-ordinates of P.
Find the equation of the line which is perpendicular to the line `x/a - y/b = 1` at the point where this line meets y-axis.
A (5, 4), B (–3,–2) and C (1,–8) are the vertices of a triangle ABC. Find the equation of median AD and line parallel to AB passing through point C.
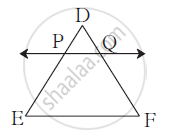
In Δ DEF, line PQ || side EF, If DP = 2.4,
PE = 7.2, DQ = 1 then find QF.
Find the equation of the line that has x-intercept = –3 and is perpendicular to 3x + 5y = 1.
Find the equation of line through the intersection of lines 2x – y = 1 and 3x + 2y = –9 and making an angle of 30° with positive direction of x-axis.
Find the equation of the line through the points A(–1, 3) and B(0, 2). Hence, show that the point A, B and C(1, 1) are collinear.
