Advertisements
Advertisements
Question
In ΔABC, A(3, 5), B(7, 8) and C(1, –10). Find the equation of the median through A.
Solution
The vertices of ΔABC are A(3, 5), B(7, 8) and C(1, –10).
Coordinates of the mid-point D of BC =
`((7 + 1)/2, (8 - 10)/2) = (8/2, (-2)/2) = (4, -1)`
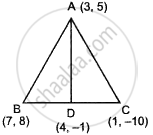
Slope of AD = `(y_2 - y_1)/(x_2 - x_1)`
= `(-1 - 5)/(4 - 3)`
= `(-6)/1`
Slope of AD = – 6
Now, the equation of median AD is given by
y – y1 = m(x – x1)
∴ y – 5 = – 6(x – 3)
∴ y – 5 = – 6x + 18
∴ 6x + y – 5 – 18 = 0
∴ 6x + y – 23 = 0
RELATED QUESTIONS
Is the line 3x + 2y = 5 parallel to the line x + 2y = 1?
B(−5, 6) and D(1, 4) are the vertices of rhombus ABCD. Find the equations of diagonals BD and AC.
The points A, B and C are (4, 0), (2, 2) and (0, 6) respectively. Find the equations of AB and BC. If AB cuts the y-axis at P and BC cuts the x-axis at Q, find the co-ordinates of P and Q.
A (5, 4), B (–3,–2) and C (1,–8) are the vertices of a triangle ABC. Find the equation of median AD and line parallel to AB passing through point C.
Verify that points P(–2, 2), Q(2, 2) and R(2, 7) are vertices of a right angled triangle.
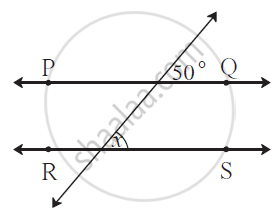
In the figure, line PQ || line RS. Using the information given
in the figure find the value of x.
In the given figure, line AB meets y-axis at point A. Line through C(2, 10) and D intersects line AB at right angle at point P. Find:
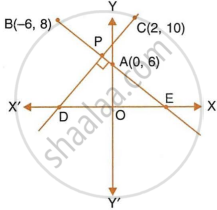
- equation of line AB.
- equation of line CD.
- co-ordinates of points E and D.
A line through point P(4, 3) meets x-axis at point A and the y-axis at point B. If BP is double of PA, find the equation of AB.
Find the equation of the line through the points A(–1, 3) and B(0, 2). Hence, show that the point A, B and C(1, 1) are collinear.
A line segment joining P(2, –3) and Q(0, –1) is cut by the x-axis at the point R. A line AB cuts the y-axis at T(0, 6) and is perpendicular to PQ at S.
Find the:
- equation of line PQ
- equation of line AB
- coordinates of points R and S.
