Advertisements
Advertisements
Question
Find the length of a solid cylinder of diameter 4 cm when recast into a hollow cylinder of outer diameter 10 cm, thickness 0.25 cm and length 21 cm? Give your answer correct to two decimal places.
Solution
For the solid cylinder:
diameter = 4cm
radius = 2cm
Let its length be l.
Volume of solid cylinder
= πr2l
= π22l
= 4πl cm3
For the hollow cylinder:
Outer diameter = 10cm
Outer radius(R) = 5cm
Inner radius(r) = R - thickness
r = 5 - 0.25
r = 4.75cm
Volume of the hollow cylinder
= πR2h - πr2h
= πh (52 - 4.752)
= π x 21(25 - 22.5625)
= 51.1875π cm3
Since the solid cylinder is recast into a hollow cylinder,
Volume of solid cylinder
= Volume of material in the hollow cylinder
4πl = 51.1875π
l = `(51.1875π)/(4π)`
l = 12.80cm
∴ The length of the solid cylinder is 12.80cm.
APPEARS IN
RELATED QUESTIONS
A swimming pool is 40 m long and 15 m wide. Its shallow and deep ends are 1.5 m and 3 m deep respectively. If the bottom of the pool slopes uniformly, find the amount of water in liters required to fill the pool.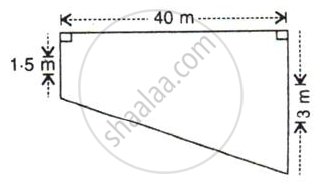
A swimming pool is 18 m long and 8 m wide. Its deep and shallow ends are 2 m and 1.2 m respectively. Find the capacity of the pool, assuming that the bottom of the pool slopes uniformly.
A school auditorium is 40 m long, 30 m broad and 12 m high. If each student requires 1.2 m2 of the floor area; find the maximum number of students that can be accommodated in this auditorium. Also, find the volume of air available in the auditorium, for each student.
The internal dimensions of a rectangular box are 12 cm x `x` cm x 9 cm. If the length of the longest rod that can be placed in this box is 17 cm; find `x`.
Find the length of 22 kg copper wire of diameter 0.8 cm, if the weight of 1 cm3 copper is 4.2 g.
The cross section of a piece of metal 2 m in length is shown. Calculate the area of cross section.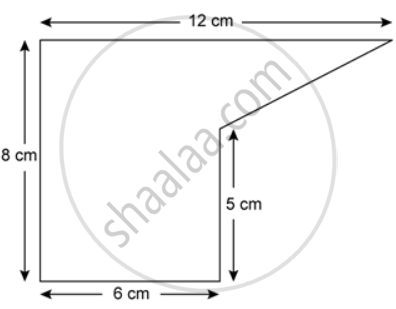
The cross section of a piece of metal 2 m in length
is shown. Calculate the volume of the piece of metal.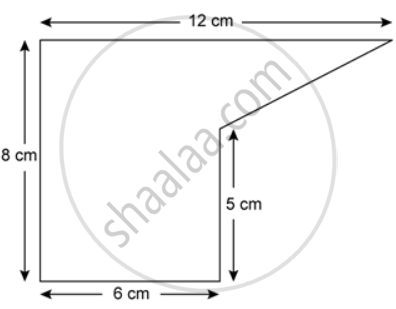
A swimming pool is 50 m long and 15 m wide. Its shallow and deep ends are 1.5 m and 4.5 m respectively. If the bottom of the pool slopes uniformly, find the amount of water in kilolitres required to fill the pool (1 m3 = 1000 liters).
The figure shows the cross section of 0.2 m a concrete wall to be constructed. It is 0.2 m wide at the top, 2.0 m wide at the bottom and its height is 4.0 m, and its length is 40 m. Calculate the cross sectional area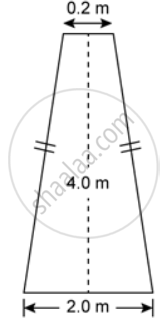
The cross section of a swimming pool is a trapezium whose shallow and deep ends are 1 m and 3 m respectively. If the length of the pool is 50 m and its width is 1.5 m, calculate the volume of water it holds.
