Advertisements
Advertisements
Question
Find the value of following determinant.
`|(5,3), (-7,0)|`
Solution
`|(5,3), (-7,0)|`
= (5 × 0) − (3 × (−7))
= 0 − (−21)
= 0 + 21
= 21
APPEARS IN
RELATED QUESTIONS
Solve the following system of equations `\frac { 1 }{ 2x } – \frac { 1 }{ y } = – 1; \frac { 1 }{ x } + \frac { 1}{ 2y } = 8`
Solve `\frac { 2 }{ x } + \frac { 1 }{ 3y } = \frac { 1}{ 5 }; \frac { 3 }{ x } + \frac { 2 }{ 3y } = 2` and also find ‘a’ for which y = ax – 2
Solve `\frac{1}{x+y}+\frac{2}{x-y}=2\text{ and }\frac{2}{x+y}-\frac{1}{x-y}=3` where, x + y ≠ 0 and x – y ≠ 0
Solve the following pairs of equations by reducing them to a pair of linear equations
`5/(x-1) + 1/y-2 = 2`
`6/(x-1) - 3/(y-2) = 1`
Solve the following pairs of equations by reducing them to a pair of linear equations
6x + 3y = 6xy
2x + 4y = 5xy
Solve the following pairs of equations by reducing them to a pair of linear equations
`10/(x+y) + 2/(x-y) = 4`
`15/(x+y) - 5/(x-y) = -2`
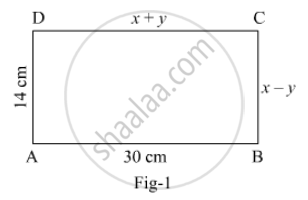
In Fig. 1, ABCD is a rectangle. Find the value of x and y.
A and B each have a certain number of mangoes. A says to B, "if you give 30 of your mangoes, I will have twice as many as left with you." B replies, "if you give me 10, I will have thrice as many as left with you." How many mangoes does each have?
A two-digit number is 4 times the sum of its digits. If 18 is added to the number, the digits are reversed. Find the number.
A two-digit number is 3 more than 4 times the sum of its digits. If 8 is added to the number, the digits are reversed. Find the number.
A two-digit number is 4 more than 6 times the sum of its digits. If 18 is subtracted from the number, the digits are reversed. Find the number.
The sum of a numerator and denominator of a fraction is 18. If the denominator is increased by 2, the fraction reduces to 1/3. Find the fraction.
The sum of the numerator and denominator of a fraction is 12. If the denominator is increased by 3, the fraction becomes 1/2. Find the fraction.
Six years hence a man's age will be three times the age of his son and three years ago he was nine times as old as his son. Find their present ages.
Ten years ago, a father was twelve times as old as his son and ten years hence, he will be twice as old as his son will be then. Find their present ages.
Father's age is three times the sum of age of his two children. After 5 years his age will be twice the sum of ages of two children. Find the age of father.
Asha has only ₹1 and ₹2 coins with her. If the total number of coins that she has is 50 and the amount of money with her is ₹75, then the number of ₹1 and ₹2 coins are, respectively.
