Advertisements
Advertisements
Question
Find the value of x so that the line passing through (3, 4) and (x, 5) makes an angle 135° with positive direction of X-axis.
Solution
Slope of the line which makes an angle 135° with X-axis,
m = tan 135°
= -1.
Also slope m = `(5 - 4)/(x - 3) = (1)/(x - 3)`
Then, `(1)/(x - 3)` = -1
⇒ x - 3 = -1
∴ x = 2.
APPEARS IN
RELATED QUESTIONS
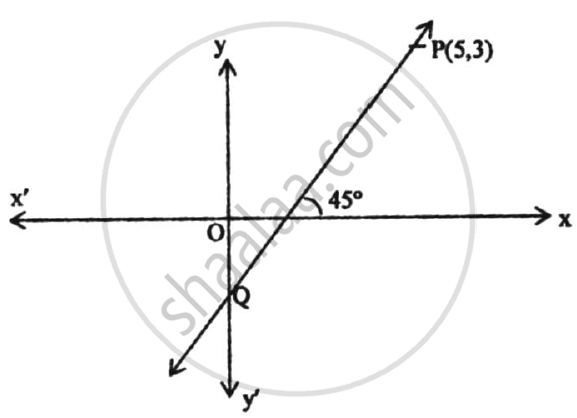
The line through P(5, 3) intersects y-axis at Q.
(1) Write the slope of the line.
(2) Write the equation of the line.
(3) Find the coordinates of Q.
Determine whether the given point is collinear.
A (0, 2), B (1, -0.5), C (2, -3)
Find k if the line passing through points P(–12, –3) and Q(4, k) has slope \[\frac{1}{2}\].
Show that A(4, –1), B(6, 0), C(7, –2) and D(5, –3) are vertices of a square.
Find the slope of a line passing through the given pair of points (0,5) and (5,0)
Write the equation of a line passing through the point P (0,6) and having slope 6/7.
Given that (a, 2a) lies on line`(y)/(2) = 3 - 6`.Find the value of a.
Find the slope of the line passing through given points G(3, 7) and K(–2, –3).
Determine whether the following points are collinear. A(–1, –1), B(0, 1), C(1, 3)
Given: Points A(–1, –1), B(0, 1) and C(1, 3)
Slope of line AB = `(square - square)/(square - square) = square/square` = 2
Slope of line BC = `(square - square)/(square - square) = square/square` = 2
Slope of line AB = Slope of line BC and B is the common point.
∴ Points A, B and C are collinear.
What is the name of the point of intersection of coordinate axes?
